|
Size: 22649
Comment:
|
Size: 24120
Comment:
|
| Deletions are marked like this. | Additions are marked like this. |
| Line 1: | Line 1: |
| see the attachments |
|
| Line 11: | Line 9: |
| = Animated = | = Animations = |
| Line 166: | Line 166: |
| '''Returns a plot of the towers of Hanoi Uses matrix_plot ''' K=max(max(l) for l in towers if l)+1 M=matrix(ZZ,K,6*K+7) #first tower |
""" Return a plot of the towers of Hanoi. This uses matrix_plot. """ K = max(max(l) for l in towers if l) + 1 M = matrix(ZZ, K, 6 * K + 7) # first tower |
| Line 175: | Line 176: |
| k=towers[0][t]-1 | k = towers[0][t] - 1 |
| Line 177: | Line 178: |
| M[K-1-j,l]=1 #second tower |
M[K-1-j,l] = 1 # second tower |
| Line 181: | Line 182: |
| k=towers[1][t]-1 | k = towers[1][t] - 1 |
| Line 183: | Line 184: |
| M[K-1-j,l]=1 #third tower |
M[K-1-j,l] = 1 # third tower |
| Line 187: | Line 188: |
| k=towers[2][t]-1 | k = towers[2][t]-1 |
| Line 189: | Line 190: |
| M[K-1-j,l]=1 | M[K-1-j,l] = 1 |
| Line 259: | Line 260: |
| = Pictures drawn by Sage = | = Pictures = |
| Line 262: | Line 263: |
== Snowman == * Fun art of spheres and cones: {{{#!python numbers=none from sage.plot.plot3d.shapes import Cone, Sphere r_bot = 3 r_mid = 2.25 r_top = 1.75 z_bot = r_bot z_mid = z_bot + r_bot + 1/2 * r_mid z_top = z_mid + r_mid + 1/2 * r_top # scale factors to shrink spheres along one axis s_body = 3/4 # vertical scale for body s_btns = 1/4 # horizontal scale for buttons s_eyes = 3/4 # horizontal scale for eyes z_bot_s = s_body * z_bot z_mid_s = s_body * z_mid z_top_s = s_body * z_top nose_length = 3/2*r_top r_button = 1/4 r_nose = 1/4 r_eye = 1/8 body_color = 'white' button_color = 'red' eye_color = 'black' nose_color = 'orange' body_bot = sphere((0, 0, z_bot), r_bot, color=body_color) body_mid = sphere((0, 0, z_mid), r_mid, color=body_color) body_top = sphere((0, 0, z_top), r_top, color=body_color) body = (body_bot + body_mid + body_top).scale(1, 1, s_body) button = Sphere(r_button, color=button_color).scale(s_btns, 1, 1) button_bot = button.translate(r_bot, 0, z_bot_s) button_mid = button.translate(r_mid, 0, z_mid_s) buttons = button_bot + button_mid eye_angle = pi/10 eye = Sphere(r_eye, color=eye_color).scale(s_eyes, 1, 1) eye = eye.translate((r_top, 0, z_top_s)) eyes = sum(eye.rotateZ(t) for t in (-eye_angle, eye_angle)) nose = Cone(r_nose, nose_length, color=nose_color) nose = nose.rotateY(-9/8*pi/2).translate(0, 0, z_top_s) parts = [body, buttons, eyes, nose] snowie = sum(parts) snowie.show(frame=False) }}} [[attachment:snowman.png|{{attachment:snowman.png||width=400}}]] |
|
| Line 354: | Line 414: |
| {{attachment:fourspheres.png}} | [[attachment:Blue_fractal_tachyon_wiki.png|{{attachment:Blue_fractal_tachyon_wiki.png||width=400}}]] |
| Line 380: | Line 440: |
| {{http://sage.math.washington.edu/home/wdj/art/padic-seasons-greetings.png}} This is the code: |
[[attachment:Blue_fractal_tachyon_wiki.png|{{attachment:Greetings_wiki.png||width=400}}]] Here is the code: |
| Line 396: | Line 456: |
| Draws Loretz butterfly using matplotlib (2d) or jmol (3d). | Draws Lorentz butterfly using matplotlib (2d) or jmol (3d). |
| Line 403: | Line 463: |
| EXAMPLE: | EXAMPLES:: |
| Line 423: | Line 484: |
| EXAMPLE: | EXAMPLES:: |
| Line 443: | Line 505: |
{{http://sage.math.washington.edu/home/wdj/art/butterfly2d.png}} |
[[attachment:Butterfly_2d_wiki.png|{{attachment:Butterfly_2d_wiki.png||width=400}}]] |
| Line 476: | Line 537: |
| {{attachment:feigenbaum.png}} |
[[attachment:feigenbaum.png|{{attachment:feigenbaum.png||width=400}}]] |
| Line 481: | Line 541: |
| * This was a black+white Sierpinski triangle coded by Marshall Hampton, with some slight tweeking by David Joyner to add colors: | * This was a black and white Sierpinski triangle coded by Marshall Hampton, with some slight tweeking by David Joyner to add colors: |
| Line 510: | Line 570: |
| GIMP was used to add a Season's greetings message: {{http://sage.math.washington.edu/home/wdj/art/sierpinski-seasons-greetings-from-sage.png}} Also (thanks to Rob Beezer) available in poster form in pdf format: http://sage.math.washington.edu/home/wdj/art/seasons-greetings-sage.pdf, and in A4 size: http://sage.math.washington.edu/home/wdj/art/seasons-greetings-sage-a4.pdf. |
[[attachment:Sierpinski_wiki.png|{{attachment:Sierpinski_wiki.png||width=400}}]] |
| Line 596: | Line 651: |
| {{attachment:Apollonian.png}} | [[attachment:Appolonian_wiki.png|{{attachment:Appolonian_wiki.png||width=400}}]] |
| Line 642: | Line 697: |
| . | [[attachment:Graph_call_wiki.png|{{attachment:Graph_call_wiki.png||width=400}}]] |
| Line 644: | Line 700: |
| #D3js interactive version (just a screenshot here) | # D3js interactive version |
| Line 646: | Line 702: |
| [edge for edge in g.edges() if edge[-1]==el] | [edge for edge in g.edges() if edge[-1] == el] |
| Line 659: | Line 715: |
| {{attachment:call_graph_partitions_js_2.png}} {{attachment:call_graph_partitions.png}} |
|
| Line 679: | Line 733: |
| very extensive plotting library with a user interface that is very similiar to Matlab's plotting. | very extensive plotting library with a user interface that is very similar to Matlab's plotting. |
| Line 682: | Line 736: |
| There are also several links to plotting/graphics/data visualization programs at the scipy [[http://www.scipy.org/Topical_Software#head-b98ffdb309ccce4e4504a25ea75b5c806e4897b6|wiki]]. | There are also several links to plotting/graphics/data visualization programs at the scipy [[https://www.scipy.org/Topical_Software#head-b98ffdb309ccce4e4504a25ea75b5c806e4897b6|wiki]]. |
This page contains animations drawn using Sage. One can create an animation (.gif) in Sage from a list of graphics objects using the animate command. Currently, to export an animation in .gif format, you might need to install the ImageMagick command line tools package (the convert command). See the documentation for more information:
sage: animate?
Contents
- Animations
-
Pictures
- Snowman
- Everywhere continuous, nowhere differentiable function
- Mirrored balls in tachyon
- Math art by Tom Boothby
- Twisted cubic in tachyon
- Reflections from four spheres in tachyon
- A cone inside a sphere
- A cylinder inside a cone
- p-adic Seasons Greetings
- Lorentz butterfly
- Feigenbaum diagram
- Sierpinski triangle
- Integral Curvature Apollonian Circle Packing
- Call graph of a recursive function
- Sage plotting
Animations
The witch of Maria Agnesi
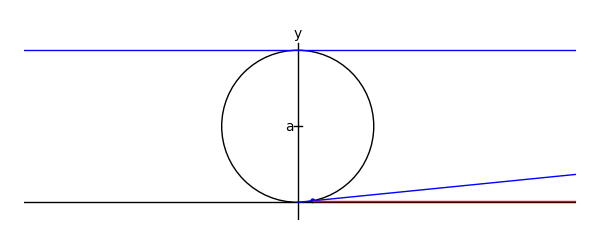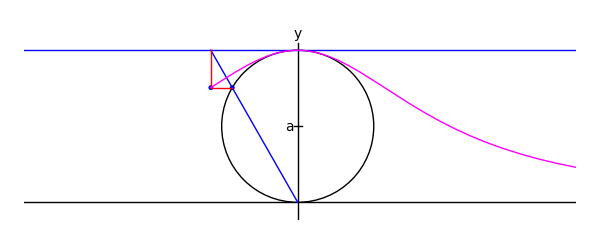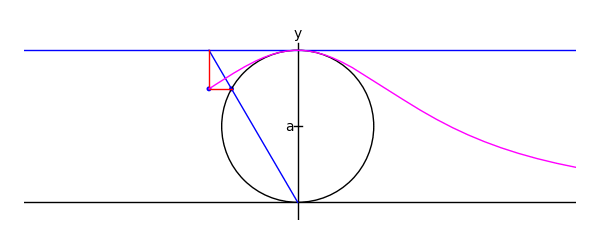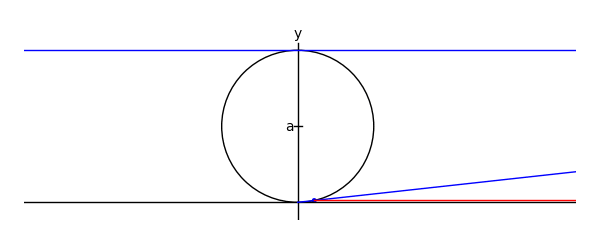
by Marshall Hampton
xtreme = 4.1
myaxes = line([[-xtreme,0],[xtreme,0]],rgbcolor = (0,0,0))
myaxes = myaxes + line([[0,-1],[0,2.1]],rgbcolor = (0,0,0))
a = 1.0
t = var('t')
npi = RDF(pi)
def agnesi(theta):
mac = circle((0,a),a,rgbcolor = (0,0,0))
maL = line([[-xtreme,2*a],[xtreme,2*a]])
maL2 = line([[0,0],[2*a*cot(theta),2*a]])
p1 = [2*a*cot(theta),2*a*sin(theta)^2]
p2 = [2*a*cot(theta)-cot(theta)*(2*a-2*a*sin(theta)^2),2*a*sin(theta)^2]
maL3 = line([p2,p1,[2*a*cot(theta),2*a]], rgbcolor = (1,0,0))
map1 = point(p1)
map2 = point(p2)
am = line([[-.05,a],[.05,a]], rgbcolor = (0,0,0))
at = text('a',[-.1,a], rgbcolor = (0,0,0))
yt = text('y',[0,2.2], rgbcolor = (0,0,0))
xt = text('x',[xtreme + .1,-.1], rgbcolor = (0,0,0))
matext = at+yt+xt
ma = mac+myaxes+maL+am+matext+maL2+map1+maL3+map2
return ma
def witchy(theta):
ma = agnesi(theta)
agplot = parametric_plot([2*a*cot(t),2*a*sin(t)^2],[t,.001,theta], rgbcolor = (1,0,1))
return ma+agplot
a2 = animate([witchy(i) for i in srange(.1,npi-.1,npi/60)]+[witchy(i) for i in srange(npi-.1,.1,-npi/60)], xmin = -3, xmax = 3, ymin = 0, ymax = 2.3, figsize = [6,2.3], axes = False)
a2.show()
A simpler hypotrochoid
The following animates a hypotrochoid
import operator
# The colors for various elements of the plot:
class color:
stylus = (1, 0, 0)
outer = (.8, .8, .8)
inner = (0, 0, 1)
plot = (0, 0, 0)
center = (0, 0, 0)
tip = (1, 0, 0)
# and the corresponding line weights:
class weight:
stylus = 1
outer = 1
inner = 1
plot = 1
center = 5
tip = 5
scale = 1 # The scale of the image
animation_delay = .1 # The delay between frames, in seconds
# Starting and ending t values
t_i = 0
t_f = 2*pi
# The t values of the animation frames
tvals = srange(t_i, t_f, (t_f-t_i)/60)
r_o = 8 # Outer circle radius
r_i = 2 # Inner circle radius
r_s = 3 # Stylus radius
# Coordinates of the center of the inner circle
x_c = lambda t: (r_o - r_i)*cos(t)
y_c = lambda t: (r_o - r_i)*sin(t)
# Parametric coordinates for the plot
x = lambda t: x_c(t) + r_s*cos(t*(r_o/r_i))
y = lambda t: y_c(t) - r_s*sin(t*(r_o/r_i))
# Maximum x and y values of the plot
x_max = r_o - r_i + r_s
y_max = find_maximum_on_interval(y, t_i, t_f)[0]
# The plots of the individual elements. Order is important; plots
# are stacked from bottom to top as they appear.
elements = (
# The outer circle
lambda t_f: circle((0, 0), r_o, rgbcolor=color.outer, thickness=weight.outer),
# The plot itself
lambda t_f: parametric_plot((x, y), t_i, t_f, rgbcolor=color.plot, thickness=weight.plot),
# The inner circle
lambda t_f: circle((x_c(t_f), y_c(t_f)), r_i, rgbcolor=color.inner, thickness=weight.inner),
# The inner circle's center
lambda t_f: point((x_c(t_f), y_c(t_f)), rgbcolor=color.center,pointsize=weight.center),
# The stylus
lambda t_f: line([(x_c(t_f), y_c(t_f)), (x(t_f), y(t_f))], rgbcolor=color.stylus, thickness=weight.stylus),
# The stylus' tip
lambda t_f: point((x_c(t_f), y_c(t_f)), rgbcolor=color.tip, pointsize=weight.tip),
)
# Create the plots and animate them. The animate function renders an
# animated gif from the frames provided as its first argument.
# Though avid python programmers will find the syntax clear, an
# explanation is provided for novices.
animation = animate([sum(f(t) for f in elements)
for t in tvals],
xmin=-x_max, xmax=x_max,
ymin=-y_max, ymax=y_max,
figsize=(x_max*scale, y_max*scale * y_max/x_max))
animation.show(delay=animation_delay)
# The previous could be expressed more pedagogically as follows:
#
# Evaluate each function in the elements array for the provided t
# value:
#
# plots = lambda t: f(t) for f in elements
#
# Join a group of plots together to form a single plot:
#
# def join_plots(plots):
# result = plots[0]
# for plot in plots[1:]:
# result += plot
# return result
#
# or
#
# join_plots = sum
#
# Create an array of plots, one for each provided t value:
#
# frames = [join_plots(plots(t)) for t in tvals]
#
# Finally, animate the frames:
#
# animation = animate(frames)
The Towers of Hanoi

by Pablo Angulo
def plot_towers(towers):
"""
Return a plot of the towers of Hanoi.
This uses matrix_plot.
"""
K = max(max(l) for l in towers if l) + 1
M = matrix(ZZ, K, 6 * K + 7)
# first tower
for t in range(len(towers[0])):
j = t
k = towers[0][t] - 1
for l in range(K+1-k,K+2+k):
M[K-1-j,l] = 1
# second tower
for t in range(len(towers[1])):
j = t
k = towers[1][t] - 1
for l in range(3*K+3-k,3*K+4+k):
M[K-1-j,l] = 1
# third tower
for t in range(len(towers[2])):
j = t
k = towers[2][t]-1
for l in range(5*K+5-k,5*K+6+k):
M[K-1-j,l] = 1
return matrix_plot(M, axes=False)
def animate_towers(towers,a=0,b=1,c=2,k=-1):
'''Move last k discs from column a into column b
Assumes that the last k discs of column a are all smaller
than the discs in columns b and c
'''
if k==0: return
if k==-1: k=len(towers[a])
for t in animate_towers(towers,a,c,b,k-1):
yield t
disc = towers[a].pop()
towers[b].append(disc)
yield plot_towers(towers)
for t in animate_towers(towers,c,b,a,k-1):
yield t
towers = (range(4,0,-1),[],[])
initial = plot_towers(towers)
frame_list=[initial]+list(animate_towers(towers))
animate(frame_list, axes=False).show(delay=80)
Fibonacci Tiles

by Sébastien Labbé
sage: path_op = dict(rgbcolor='red', thickness=1)
sage: fill_op = dict(rgbcolor='blue', alpha=0.3)
sage: options = dict(pathoptions=path_op, filloptions=fill_op, endarrow=False, startpoint=False)
sage: G = [words.fibonacci_tile(i).plot(**options) for i in range(7)]
sage: a = animate(G)
sage: a.show(delay=150)
Pencil of conics
by Pablo Angulo 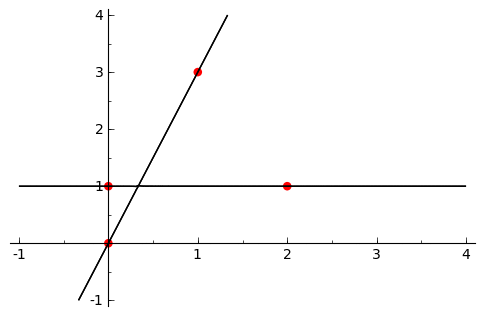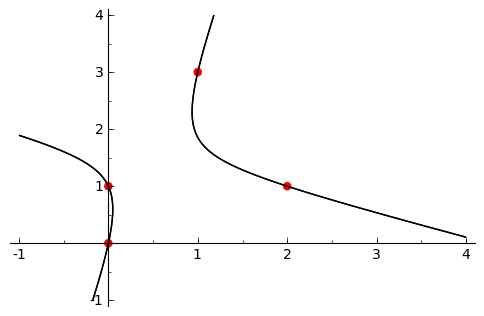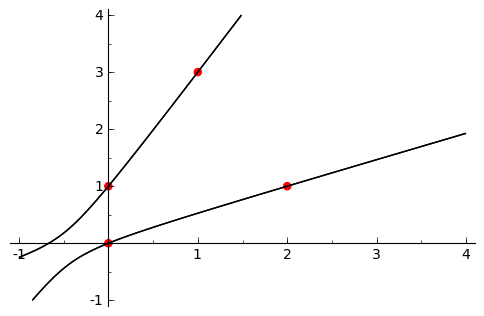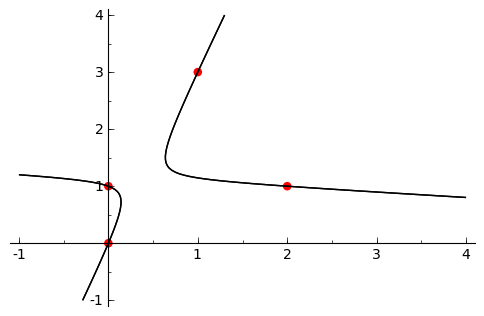
puntos = [(0,0),(0,1),(1,3),(2,1)]
K = len(puntos)
var('x y')
coefs = matrix(QQ, K, 6)
for j in range(K):
x0, y0 = puntos[j]
coefs[j,:] = vector([x0^2, y0^2, x0*y0, x0, y0, 1])
K = coefs.right_kernel()
v1 = K.basis()[0]
v2 = K.basis()[1]
graficas = []
for t in srange(0,2*pi,0.3):
c1, c2 = sin(t), cos(t)
a,b,c,d,e,f = c1*v1 + c2*v2
curva = a*x^2 + b*y^2 + c*x*y + d*x + e*y + f
graficas.append( point2d(puntos,color=(1,0,0),pointsize=30) +
implicit_plot(curva,(x,-1,4),(y,-1,4)) )
a = animate(graficas)
a.show(delay=10)
Pictures
These pictures and images were drawn by Sage.
Snowman
- Fun art of spheres and cones:
from sage.plot.plot3d.shapes import Cone, Sphere
r_bot = 3
r_mid = 2.25
r_top = 1.75
z_bot = r_bot
z_mid = z_bot + r_bot + 1/2 * r_mid
z_top = z_mid + r_mid + 1/2 * r_top
# scale factors to shrink spheres along one axis
s_body = 3/4 # vertical scale for body
s_btns = 1/4 # horizontal scale for buttons
s_eyes = 3/4 # horizontal scale for eyes
z_bot_s = s_body * z_bot
z_mid_s = s_body * z_mid
z_top_s = s_body * z_top
nose_length = 3/2*r_top
r_button = 1/4
r_nose = 1/4
r_eye = 1/8
body_color = 'white'
button_color = 'red'
eye_color = 'black'
nose_color = 'orange'
body_bot = sphere((0, 0, z_bot), r_bot, color=body_color)
body_mid = sphere((0, 0, z_mid), r_mid, color=body_color)
body_top = sphere((0, 0, z_top), r_top, color=body_color)
body = (body_bot + body_mid + body_top).scale(1, 1, s_body)
button = Sphere(r_button, color=button_color).scale(s_btns, 1, 1)
button_bot = button.translate(r_bot, 0, z_bot_s)
button_mid = button.translate(r_mid, 0, z_mid_s)
buttons = button_bot + button_mid
eye_angle = pi/10
eye = Sphere(r_eye, color=eye_color).scale(s_eyes, 1, 1)
eye = eye.translate((r_top, 0, z_top_s))
eyes = sum(eye.rotateZ(t) for t in (-eye_angle, eye_angle))
nose = Cone(r_nose, nose_length, color=nose_color)
nose = nose.rotateY(-9/8*pi/2).translate(0, 0, z_top_s)
parts = [body, buttons, eyes, nose]
snowie = sum(parts)
snowie.show(frame=False)
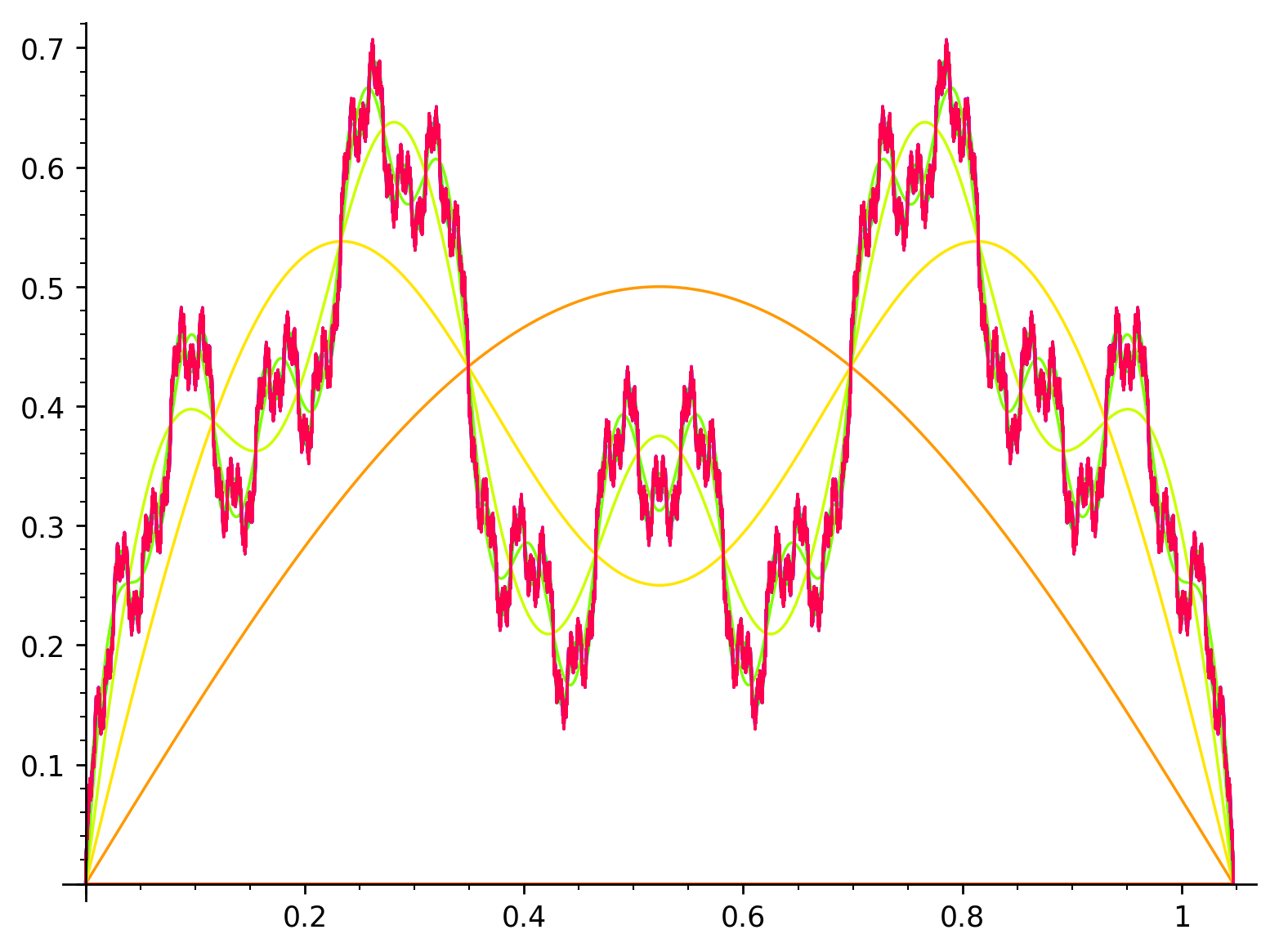
Everywhere continuous, nowhere differentiable function
- Everywhere continuous, nowhere differentiable function (in the infinite limit, anyway):
p = Graphics()
for n in range(1,20):
f = lambda x: sum([sin(x*3^i)/(2^i) for i in range(1,n)])
p += plot(f,0,float(pi/3),plot_points=2000,rgbcolor=hue(n/20))
p.show(xmin=0, ymin=0,dpi=250)
Mirrored balls in tachyon
t = Tachyon(camera_center=(8.5,5,5.5), look_at=(2,0,0), raydepth=6, xres=1500, yres=1500)
t.light((10,3,4), 1, (1,1,1))
t.texture('mirror', ambient=0.05, diffuse=0.05, specular=.9, opacity=0.9, color=(.8,.8,.8))
t.texture('grey', color=(.8,.8,.8), texfunc=7) ## try other values of texfunc too!
t.plane((0,0,0),(0,0,1),'grey')
t.sphere((4,-1,1), 1, 'mirror')
t.sphere((0,-1,1), 1, 'mirror')
t.sphere((2,-1,1), 0.5, 'mirror')
t.sphere((2,1,1), 0.5, 'mirror')
show(t)
Math art by Tom Boothby
# Author: Tom Boothby
# This is a remake of an old art piece I made in POVRay
t = Tachyon(xres=1000,yres=600, camera_center=(1,0,5), antialiasing=3)
t.light((4,3,2), 0.2, (1,1,1))
t.texture('t0', ambient=0.1, diffuse=0.9, specular=0.5, opacity=1.0, color=(1.0,1,1))
t.texture('t1', ambient=0.5, diffuse=0.5, specular=0.0, opacity=1.0, color=(0,0,0))
t.texture('t2', ambient=0.2, diffuse=0.7, specular=0, opacity=0.7, color=(.5,.5,.5))
t.texture('t3', ambient=.9, diffuse=5, specular=0,opacity=.1, color=(1,0,0))
t.sphere((1,0,0), 30, 't2')
k=0
for i in srange(-pi*10,0,.01):
k += 1
t.sphere((cos(i/10)-.1, sin(i/10)*cos(i), sin(i/10)*sin(i)), 0.1, 't0')
t.sphere((cos(i/10) + 2.1, sin(i/10)*cos(i), sin(i/10)*sin(i)), 0.1, 't1')
t.show(verbose=1)
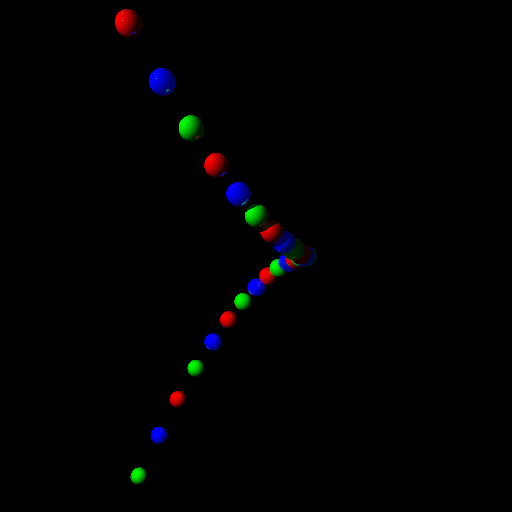
Twisted cubic in tachyon
t = Tachyon(xres=512,yres=512, camera_center=(5,0,0))
t.light((4,3,2), 0.2, (1,1,1))
t.texture('t0', ambient=0.1, diffuse=0.9, specular=0.5, opacity=1.0, color=(1.0,0,0))
t.texture('t1', ambient=0.1, diffuse=0.9, specular=0.3, opacity=1.0, color=(0,1.0,0))
t.texture('t2', ambient=0.2, diffuse=0.7, specular=0.5, opacity=0.7, color=(0,0,1.0))
k=0
for i in srange(-5,1.5,0.1):
k += 1
t.sphere((i,i^2-0.5,i^3), 0.1, 't%s'%(k%3))
t.show()
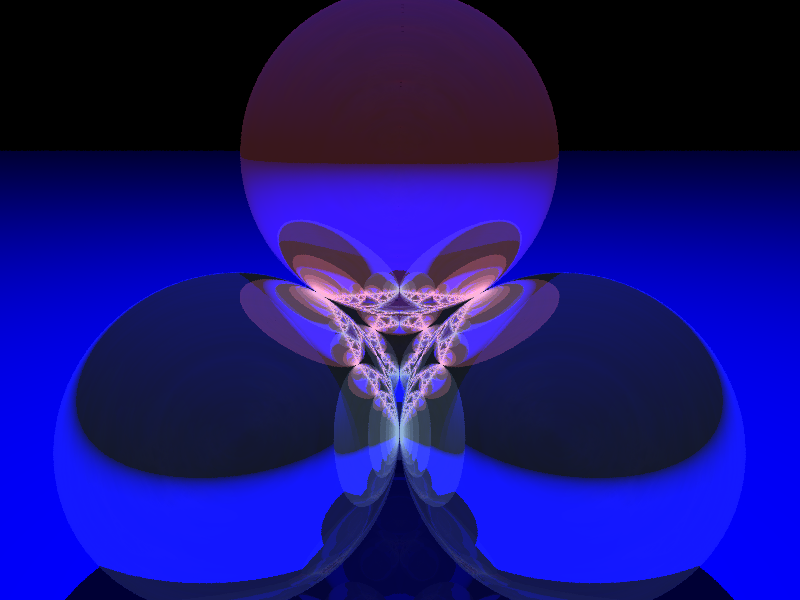
Reflections from four spheres in tachyon
t6 = Tachyon(camera_center=(0,-4,1), xres = 800, yres = 600, raydepth = 12, aspectratio=.75, antialiasing = True)
t6.light((0.02,0.012,0.001), 0.01, (1,0,0))
t6.light((0,0,10), 0.01, (0,0,1))
t6.texture('s', color = (.8,1,1), opacity = .9, specular = .95, diffuse = .3, ambient = 0.05)
t6.texture('p', color = (0,0,1), opacity = 1, specular = .2)
t6.sphere((-1,-.57735,-0.7071),1,'s')
t6.sphere((1,-.57735,-0.7071),1,'s')
t6.sphere((0,1.15465,-0.7071),1,'s')
t6.sphere((0,0,0.9259),1,'s')
t6.plane((0,0,-1.9259),(0,0,1),'p')
t6.show()
A cone inside a sphere
sage: u,v = var("u,v")
sage: p1 = parametric_plot3d([cos(u)*v, sin(u)*v, 3*v/2-1/3], (u, 0, 2*pi), (v, 0, 0.95),plot_points=[20,20])
sage: p2 = sphere((0,0,2/3), color='red', opacity=0.5, aspect_ratio=[1,1,1])
sage: show(p1+p2)

A cylinder inside a cone
sage: u,v = var("u,v")
sage: p1 = parametric_plot3d([cos(u)*v, sin(u)*v, 3/2-3*v/2], (u, 0, 2*pi), (v, 0, 1.5), opacity = 0.5, plot_points=[20,20])
sage: p2 = parametric_plot3d([cos(u)/2, sin(u)/2, v-3/4], (u, 0, 2*pi), (v, 0, 3/2), plot_points=[20,20])
sage: show(p1+p2)

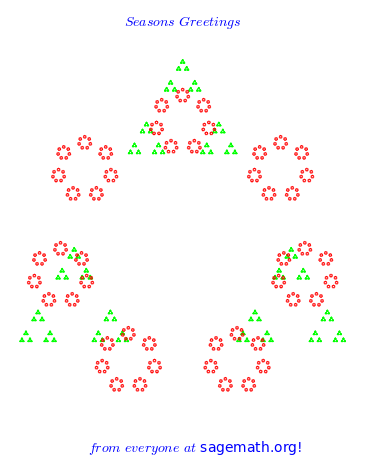
p-adic Seasons Greetings
- I know this is early, but thanks to Robert Bradshaw's p-adic plot function, here is a p-adic Seasons Greetings:
Here is the code:
sage: P1 = Zp(3).plot(rgbcolor=(0,1,0))
sage: P2 = Zp(7).plot(rgbcolor=(1,0,0))
sage: P3 = text("$Seasons$ $Greetings$ ",(0.0,1.8))
sage: P4 = text("$from$ $everyone$ $at$ sagemath.org!",(0.1,-1.6))
sage: (P1+P2+P3+P4).show(axes=False)
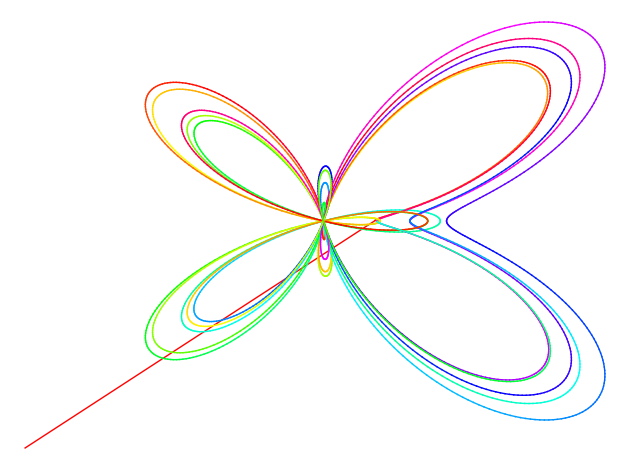
Lorentz butterfly
"""
Draws Lorentz butterfly using matplotlib (2d) or jmol (3d).
Written by Matthew Miller and William Stein.
"""
def butterfly2d():
""""
EXAMPLES::
sage: butterfly2d()
"""
g = Graphics()
x1, y1 = 0, 0
from math import sin, cos, exp, pi
for theta in srange( 0, 10*pi, 0.01 ):
r = exp(cos(theta)) - 2*cos(4*theta) + sin(theta/12)^5
x = r * cos(theta) # Convert polar to rectangular coordinates
y = r * sin(theta)
xx = x*6 + 25 # Scale factors to enlarge and center the curve.
yy = y*6 + 25
if theta != 0:
l = line( [(x1, y1), (xx, yy)], rgbcolor=hue(theta/7 + 4) )
g = g + l
x1, y1 = xx, yy
g.show(dpi=100, axes=False)
def butterfly3d():
""""
EXAMPLES::
sage: butterfly3d()
"""
g = point3d((0,0,0))
x1, y1 = 0, 0
from math import sin, cos, exp, pi
for theta in srange( 0, 10*pi, 0.05):
r = exp(cos(theta)) - 2*cos(4*theta) + sin(theta/12)^5
x = r * cos(theta) # Convert polar to rectangular coordinates
y = r * sin(theta)
xx = x*6 + 25 # Scale factors to enlarge and center the curve.
yy = y*6 + 25
if theta != 0:
l = line3d( [(x1, y1, theta), (xx, yy, theta)],
rgbcolor=hue(theta/7 + 4) )
g = g + l
x1, y1 = xx, yy
g.show(dpi=100, axes=False)

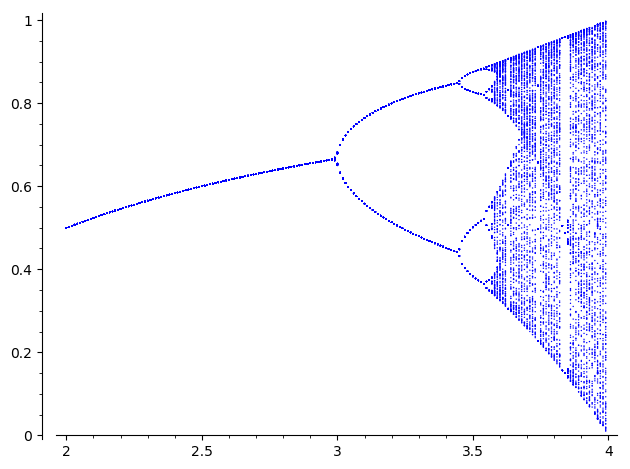
Feigenbaum diagram
Author: Pablo Angulo Posted to sage-devel 2008-09-13. See also https://sage.math.washington.edu:8101/home/pub/3 #Note: Mandelbrot set moved to interact/fractals
#Plots Feigenbaum diagram: divides the parameter interval [2,4] for mu
#into N steps. For each value of the parameter, iterate the discrete
#dynamical system x->mu*x*(1-x), drop the first M1 points in the orbit
#and plot the next M2 points in a (mu,x) diagram
N=200
M1=200
M2=200
x0=0.509434
puntos=[]
for t in range(N):
mu=2.0+2.0*t/N
x=x0
for i in range(M1):
x=mu*x*(1-x)
for i in range(M2):
x=mu*x*(1-x)
puntos.append((mu,x))
point(puntos,pointsize=1)
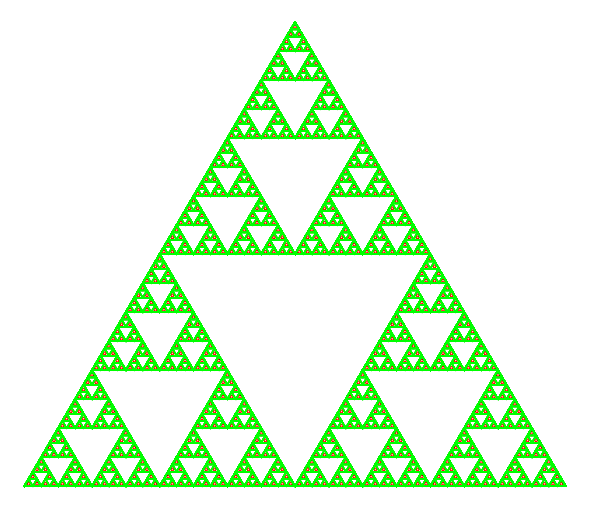
Sierpinski triangle
- This was a black and white Sierpinski triangle coded by Marshall Hampton, with some slight tweeking by David Joyner to add colors:
def sierpinski_seasons_greetings():
"""
Code by Marshall Hampton.
Colors by David Joyner.
General depth by Rob Beezer.
Copyright Marshall Hampton 2008, licensed
creative commons, attribution share-alike.
"""
depth = 7
nsq = RR(3^(1/2))/2.0
tlist_old = [[[-1/2.0,0.0],[1/2.0,0.0],[0.0,nsq]]]
tlist_new = tlist_old[:]
for ind in range(depth):
for tri in tlist_old:
for p in tri:
new_tri = [[(p[0]+x[0])/2.0, (p[1]+x[1])/2.0] for x in tri]
tlist_new.append(new_tri)
tlist_old = tlist_new[:]
T = tlist_old
N = 4^depth
N1 = N - 3^depth
q1 = sum([line(T[i]+[T[i][0]], rgbcolor = (0,1,0)) for i in range(N1)])
q2 = sum([line(T[i]+[T[i][0]], rgbcolor = (1,0,0)) for i in range(N1,N)])
show(q2+q1, figsize = [6,6*nsq], axes = False)
Integral Curvature Apollonian Circle Packing
by Marshall Hampton and Carl Witty
def kfun(k1,k2,k3,k4):
"""
The Descartes formula for the curvature of an inverted tangent circle.
"""
return 2*k1+2*k2+2*k3-k4
colorlist = [(1,0,1),(0,1,0),(0,0,1),(1,0,0)]
def circfun(c1,c2,c3,c4):
"""
Computes the inversion of circle 4 in the first three circles.
"""
newk = kfun(c1[3],c2[3],c3[3],c4[3])
newx = (2*c1[0]*c1[3]+2*c2[0]*c2[3]+2*c3[0]*c3[3]-c4[0]*c4[3])/newk
newy = (2*c1[1]*c1[3]+2*c2[1]*c2[3]+2*c3[1]*c3[3]-c4[1]*c4[3])/newk
newcolor = c4[4]
if newk > 0:
newr = 1/newk
elif newk < 0:
newr = -1/newk
else:
newr = Infinity
return [newx, newy, newr, newk, newcolor]
def mcircle(circdata, label = False, thick = 1/10, cutoff = 2000, color = ''):
"""
Draws a circle from the data. label = True
"""
if color == '':
color = colorlist[circdata[4]]
if label==True and circdata[3] > 0 and circdata[2] > 1/cutoff:
lab = text(str(circdata[3]),(circdata[0],circdata[1]), fontsize = \
500*(circdata[2])^(.95), vertical_alignment = 'center', horizontal_alignment \
= 'center', rgbcolor = (0,0,0),zorder=10)
else:
lab = Graphics()
circ = circle((circdata[0],circdata[1]), circdata[2], rgbcolor = (0,0,0), \
thickness = thick)
circ = circ + circle((circdata[0],circdata[1]), circdata[2], rgbcolor = color, \
thickness = thick, fill=True, alpha = .4, zorder=0)
return lab+circ
def add_circs(c1, c2, c3, c4, cutoff = 300):
"""
Find the inversion of c4 through c1,c2,c3. Add the result to circlist,
then (if the result is big enough) recurse.
"""
newcirc = circfun(c1, c2, c3, c4)
if newcirc[3] < cutoff:
circlist.append(newcirc)
add_circs(newcirc, c1, c2, c3, cutoff = cutoff)
add_circs(newcirc, c2, c3, c1, cutoff = cutoff)
add_circs(newcirc, c3, c1, c2, cutoff = cutoff)
zst1 = [0,0,1/2,-2,0]
zst2 = [1/6,0,1/3,3,1]
zst3 = [-1/3,0,1/6,6,2]
zst4 = [-3/14,2/7,1/7,7,3]
circlist = [zst1,zst2,zst3,zst4]
add_circs(zst1,zst2,zst3,zst4,cutoff = 500)
add_circs(zst2,zst3,zst4,zst1,cutoff = 500)
add_circs(zst3,zst4,zst1,zst2,cutoff = 500)
add_circs(zst4,zst1,zst2,zst3,cutoff = 500)
circs = sum([mcircle(q, label = True, thick = 1/2) for q in \
circlist[1:]])
circs = circs + mcircle(circlist[0],color=(1,1,1),thick=1)
circs.save('./Apollonian3.png',axes = False, figsize = [12,12], xmin = \
-1/2, xmax = 1/2, ymin = -1/2, ymax = 1/2)
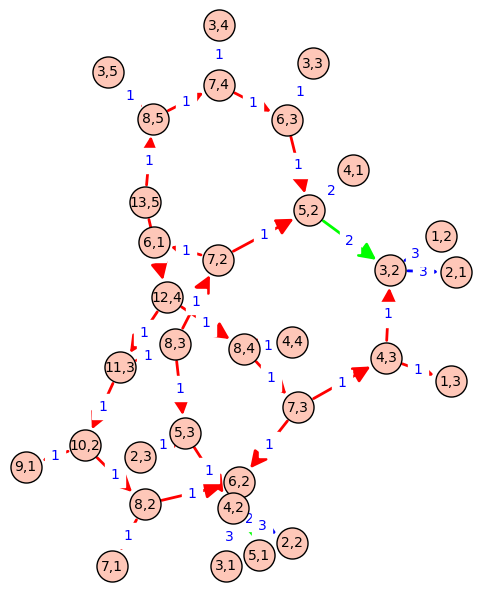
Call graph of a recursive function
def grafo_llamadas(f):
class G(object):
def __init__(self, f):
self.f=f
self.stack = []
self.g = DiGraph()
def __call__(self, *args):
if self.stack:
sargs = ','.join(str(a) for a in args)
last = ','.join(str(a) for a in self.stack[-1])
if self.g.has_edge(last, sargs):
l = self.g.edge_label(last, sargs)
self.g.set_edge_label(last, sargs, l + 1)
else:
self.g.add_edge(last, sargs, 1)
else:
self.g = DiGraph()
self.stack.append(args)
v = self.f(*args)
self.stack.pop()
return v
def grafo(self):
return self.g
return G(f)
@grafo_llamadas
def particiones(n, k):
if k == n:
return [[1]*n]
if k == 1:
return [[n]]
if not(0 < k < n):
return []
ls1 = [p+[1] for p in particiones(n-1, k-1)]
ls2 = [[parte+1 for parte in p] for p in particiones(n-k, k)]
return ls1 + ls2
particiones(13,5)
g = particiones.grafo()
g.show(edge_labels=True, figsize=(6,6), vertex_size=500, color_by_label=True)# D3js interactive version
edge_partition = [
[edge for edge in g.edges() if edge[-1] == el]
for el in set(g.edge_labels())
]
g.show(method='js',
edge_labels=True,
vertex_labels=True,
link_distance=90,
charge=-400,
link_strength=2,
force_spring_layout=True,
edge_partition=edge_partition)
Sage plotting
Here are some python plotting engines/libraries:
- Older/not python dedicated:
Grace: grace, python interface
PLplot: http://www.plplot.org
opemath: Written by William Schelter and part of Maxima (thus also Sage) is a TCL/Tk plotting program which allows for interactive viewing. It has no separate download page. An example is this saddle: sage: maxima.eval("plot3d(2^(-u^2+v^2),[u,-1,1],[v,-1,1],[plot_format, openmath]);")
Dislin: dislin, disipyl (a python wrapper for dislin). It's license says dislin is "free for non-commercial use".
Pyqwt at http://pyqwt.sourceforge.net/ is a plotting package requiring QT. It seems to have some 3d capabilities http://pyqwt.sourceforge.net/pyqwt3d-examples.html.
matplotlib: http://matplotlib.sourceforge.net
Sage's plotting functionality is built on top of matplotlib, which is a very extensive plotting library with a user interface that is very similar to Matlab's plotting. The interface that Sage provides to matplotlib is very Mathematica like.
There are also several links to plotting/graphics/data visualization programs at the scipy wiki.