Differences between revisions 44 and 84 (spanning 40 versions)
⇤ ← Revision 44 as of 2012-03-16 06:46:28 →
Size: 57805
Editor: jason
Comment:
← Revision 84 as of 2017-03-18 14:37:51 → ⇥
Size: 63362
Editor: mforets
Comment: fix DeprecationWarning in Root Finding Using Bisection
Deletions are marked like this. Additions are marked like this.
Line 6: Line 6:
{{{#!html
<script type="text/javascript" src="http://aleph.sagemath.org/static/jquery.min.js"></script>
<script type="text/javascript" src="http://aleph.sagemath.org/embedded_sagecell.js"></script>
    <script>
$(function() {
    var makecells = function() {
        sagecell.makeSagecell({
            inputLocation: '#interact1',
            evalButtonText: 'Interact'});
    }
    sagecell.init(makecells);
})</script>

}}}
Line 24: Line 10:
{{{#!html
<div id="interact1"><script type="text/code">		 {{{#!sagecell
Line 44: Line 29:
html("<h1>Double Precision Root Finding Using Bisection</h1>")
@interact
def _(f = cos(x) - x, a = float(0), b = float(1), eps=(-3,(-16..-1))):		 pretty_print(html("<h1>Double Precision Root Finding Using Bisection</h1>"))
@interact
def _(f = cos(x) - x, a = float(0), b = float(1), eps=(-3,[-16..-1])):
Line 50: Line 35:
         time c, intervals = bisect_method(f, a, b, eps)          c, intervals = bisect_method(f, a, b, eps)
Line 56: Line 41:
         print "f(c) = %r"%f(c)          print "f(c) = %r"%f(x=c)
Line 64: Line 49:
</script></div>
Line 73: Line 57:
http://sagenb.org/home/pub/2824/

{{{		 https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2824-Double%20Precision%20Root%20Finding%20Using%20Newton's%20Method.sagews

{{{#!sagecell
Line 93: Line 77:
html("<h1>Double Precision Root Finding Using Newton's Method</h1>") pretty_print(html("<h1>Double Precision Root Finding Using Newton's Method</h1>"))
Line 98: Line 82:
     time z, iterates = newton_method(f, c, eps)      z, iterates = newton_method(f, c, eps)
Line 103: Line 87:
     html(iterates)      pretty_print(html(iterates))
Line 115: Line 99:
http://sagenb.org/home/pub/2823/

{{{		 https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2823.sagews

{{{#!sagecell
Line 133: Line 117:
{{{
html('<h2>Tangent line grapher</h2>')		 {{{#!sagecell
pretty_print(html('<h2>Tangent line grapher</h2>'))
Line 145: Line 129:
    fmax = f.find_maximum_on_interval(prange[0], prange[1])[0]
    fmin = f.find_minimum_on_interval(prange[0], prange[1])[0]		     fmax = f.find_local_maximum(prange[0], prange[1])[0]
    fmin = f.find_local_minimum(prange[0], prange[1])[0]
Line 153: Line 137:
{{{ {{{#!sagecell
#find_maximum_on_interval and find_minimum_on_interval are deprecated
#use find_local_maximum find_local_minimum instead
#see http://trac.sagemath.org/2607 for details -RRubalcaba
Line 168: Line 156:
    min_y = find_minimum_on_interval(func,a,b)[0]
    max_y = find_maximum_on_interval(func,a,b)[0]
    html('<h3>Numerical integrals with the midpoint rule</h3>')
    html('$\int_{a}^{b}{f(x) dx} {\\approx} \sum_i{f(x_i) \Delta x}$')		     min_y = min(0, sage.numerical.optimize.find_local_minimum(func,a,b)[0])
    max_y = max(0, sage.numerical.optimize.find_local_maximum(func,a,b)[0])
    pretty_print(html('<h3>Numerical integrals with the midpoint rule</h3>'))
    pretty_print(html('$\int_{a}^{b}{f(x) dx} {\\approx} \sum_i{f(x_i) \Delta x}$'))
Line 181: Line 169:
{{{ {{{#!sagecell
Line 183: Line 171:
#find_maximum_on_interval and find_minimum_on_interval are deprecated
#use find_local_maximum find_local_minimum instead
#see http://trac.sagemath.org/2607 for details -RRubalcaba
Line 206: Line 197:
            x = find_maximum_on_interval(func, q*dx + a, q*dx + dx + a)[1]             x = find_local_maximum(func, q*dx + a, q*dx + dx + a)[1]
Line 209: Line 200:
            x = find_minimum_on_interval(func, q*dx + a, q*dx + dx + a)[1]             x = find_local_minimum(func, q*dx + a, q*dx + dx + a)[1]
Line 220: Line 211:
    min_y = min(0, find_minimum_on_interval(func,a,b)[0])
    max_y = max(0, find_maximum_on_interval(func,a,b)[0])		     min_y = min(0, find_local_minimum(func,a,b)[0])
    max_y = max(0, find_local_maximum(func,a,b)[0])
Line 254: Line 245:
{{{ {{{#!sagecell
Line 258: Line 249:
    html('$r=' + latex(b+sin(a1*t)^n1 + cos(a2*t)^n2)+'$')     pretty_print(html('$r=' + latex(b+sin(a1*t)^n1 + cos(a2*t)^n2)+'$'))
Line 267: Line 258:
{{{ {{{#!sagecell
Line 345: Line 336:
    html('<center><font color="red">$f = %s$</font></center>'%latex(f))
    html('<center><font color="green">$g = %s$</font></center>'%latex(g))
    html('<center><font color="blue"><b>$h = %s = %s$</b></font></center>'%(lbl, latex(h)))		     pretty_print(html('<center><font color="red">$f = %s$</font></center>'%latex(f)))
    pretty_print(html('<center><font color="green">$g = %s$</font></center>'%latex(g)))
    pretty_print(html('<center><font color="blue"><b>$h = %s = %s$</b></font></center>'%(lbl, latex(h))))
Line 365: Line 356:
{{{ {{{#!sagecell
Line 467: Line 458:
== Coordinate Transformations == == Coordinate Transformations (FIXME in Jupyter) ==
Line 471: Line 462:
{{{ {{{#!sagecell
Line 473: Line 464:
# polar coordinates
#(x,y)=(u*cos(v),u*sin(v)); (u_range,v_range)=([0..6],[0..2*pi,step=pi/12])

# weird example
(x,y)=(u^2-v^2,u*v+cos(u*v)); (u_range,v_range)=([-5..5],[-5..5])

thickness=4
square_length=.05
Line 476: Line 476:
def trans(x=input_box(u^2-v^2, label="x=",type=SR), \
         y=input_box(u*v+cos(u*v), label="y=",type=SR), \
         t_val=slider(0,10,0.2,6, label="Length of curves"), \		 def trans(x=input_box(x, label="x",type=SR),
         y=input_box(y, label="y",type=SR),
Line 481: Line 480:
         u_range=input_box(range(-5,5,1), label="u lines"),
         v_range=input_box(range(-5,5,1), label="v lines")):
     thickness=4
     u_val = min(u_range)+(max(u_range)-min(u_range))*u_percent
     v_val = min(v_range)+(max(v_range)-min(v_range))*v_percent
     t_min = -t_val
     t_max = t_val
     g1=sum([parametric_plot((i,v), (v,t_min,t_max), rgbcolor=(1,0,0)) for i in u_range])
     g2=sum([parametric_plot((u,i), (u,t_min,t_max), rgbcolor=(0,0,1)) for i in v_range])
     vline_straight=parametric_plot((u,v_val), (u,t_min,t_max), rgbcolor=(0,0,1), linestyle='-',thickness=thickness)
     uline_straight=parametric_plot((u_val, v), (v,t_min,t_max),rgbcolor=(1,0,0), linestyle='-',thickness=thickness)
 
     (g1+g2+vline_straight+uline_straight).save("uv_coord.png",aspect_ratio=1, figsize=[5,5], axes_labels=['$u$','$v$'])
     xuv = fast_float(x,'u','v')
     yuv = fast_float(y,'u','v')
     xvu = fast_float(x,'v','u')
     yvu = fast_float(y,'v','u')
     g3=sum([parametric_plot((partial(xuv,i),partial(yuv,i)), (v,t_min,t_max), rgbcolor=(1,0,0)) for i in u_range])
     g4=sum([parametric_plot((partial(xvu,i),partial(yvu,i)), (u,t_min,t_max), rgbcolor=(0,0,1)) for i in v_range])
     uline=parametric_plot((partial(xuv,u_val),partial(yuv,u_val)),(v,t_min,t_max),rgbcolor=(1,0,0), linestyle='-',thickness=thickness)
     vline=parametric_plot((partial(xvu,v_val),partial(yvu,v_val)), (u,t_min,t_max), rgbcolor=(0,0,1), linestyle='-',thickness=thickness)
     (g3+g4+vline+uline).save("xy_coord.png", aspect_ratio=1, figsize=[5,5], axes_labels=['$x$','$y$'])
     print jsmath("x=%s, \: y=%s"%(latex(x), latex(y)))
     print "<html><table><tr><td><img src='cell://uv_coord.png'/></td><td><img src='cell://xy_coord.png'/></td></tr></table></html>"		          t_val=slider(0,10,0.2,6, label="Length"),
         u_range=input_box(u_range, label="u lines"),
         v_range=input_box(v_range, label="v lines")):

    x(u,v)=x
    y(u,v)=y
    u_val = min(u_range)+(max(u_range)-min(u_range))*u_percent
    v_val = min(v_range)+(max(v_range)-min(v_range))*v_percent
    t_min = -t_val
    t_max = t_val
    uvplot=sum([parametric_plot((i,v), (v,t_min,t_max), color='red',axes_labels=['u','v'],figsize=[5,5]) for i in u_range])
    uvplot+=sum([parametric_plot((u,i), (u,t_min,t_max), color='blue',axes_labels=['u','v']) for i in v_range])
    uvplot+=parametric_plot((u,v_val), (u,t_min,t_max), rgbcolor=(0,0,1), linestyle='-',thickness=thickness)
    uvplot+=parametric_plot((u_val, v), (v,t_min,t_max),rgbcolor=(1,0,0), linestyle='-',thickness=thickness)
    pt=vector([u_val,v_val])
    du=vector([(t_max-t_min)*square_length,0])
    dv=vector([0,(t_max-t_min)*square_length])
    uvplot+=polygon([pt,pt+dv,pt+du+dv,pt+du],color='purple',alpha=0.7)
    uvplot+=line([pt,pt+dv,pt+du+dv,pt+du],color='green')

    T(u,v)=(x,y)
    xuv = fast_float(x,'u','v')
    yuv = fast_float(y,'u','v')
    xvu = fast_float(x,'v','u')
    yvu = fast_float(y,'v','u')
    xyplot=sum([parametric_plot((partial(xuv,i),partial(yuv,i)), (v,t_min,t_max), color='red', axes_labels=['x','y'],figsize=[5,5]) for i in u_range])
    xyplot+=sum([parametric_plot((partial(xvu,i),partial(yvu,i)), (u,t_min,t_max), color='blue') for i in v_range])
    xyplot+=parametric_plot((partial(xuv,u_val),partial(yuv,u_val)),(v,t_min,t_max),color='red', linestyle='-',thickness=thickness)
    xyplot+=parametric_plot((partial(xvu,v_val),partial(yvu,v_val)), (u,t_min,t_max), color='blue', linestyle='-',thickness=thickness)
    jacobian=abs(T.diff().det()).simplify_full()
    t_vals=[0..1,step=t_val*.01]
    vertices=[(x(*c),y(*c)) for c in [pt+t*dv for t in t_vals]]
    vertices+=[(x(*c),y(*c)) for c in [pt+dv+t*du for t in t_vals]]
    vertices+=[(x(*c),y(*c)) for c in [pt+(1-t)*dv+du for t in t_vals]]
    vertices+=[(x(*c),y(*c)) for c in [pt+(1-t)*du for t in t_vals]]
    xyplot+=polygon(vertices,color='purple',alpha=0.7)
    xyplot+=line(vertices,color='green')
    pretty_print(html("$T(u,v)=%s$"%(latex(T(u,v)))))
    pretty_print(html("Jacobian: $%s$"%latex(jacobian(u,v))))
    pretty_print(html("A very small region in $xy$ plane is approximately %0.4g times the size of the corresponding region in the $uv$ plane"%jacobian(u_val,v_val).n()))
    pretty_print(table([[uvplot,xyplot]]))
Line 513: Line 529:
{{{ {{{#!sagecell
Line 523: Line 539:
    html('$f(x)\;=\;%s$'%latex(f))
    html('$\hat{f}(x;%s)\;=\;%s+\mathcal{O}(x^{%s})$'%(x0,latex(ft),order+1))		     pretty_print(html('$f(x)\;=\;%s$'%latex(f)))
    pretty_print(html('$\hat{f}(x;%s)\;=\;%s+\mathcal{O}(x^{%s})$'%(x0,latex(ft),order+1)))
Line 536: Line 552:
{{{
html("<h2>Limits: <i>ε-δ</i></h2>")
html("This allows you to estimate which values of <i>δ</i> guarantee that <i>f</i> is within <i>ε</i> units of a limit.")
html("<ul><li>Modify the value of <i>f</i> to choose a function.</li>")
html("<li>Modify the value of <i>a</i> to change the <i>x</i>-value where the limit is being estimated.</li>")
html("<li>Modify the value of <i>L</i> to change your guess of the limit.</li>")
html("<li>Modify the values of <i>δ</i> and <i>ε</i> to modify the rectangle.</li></ul>")
html("If the blue curve passes through the pink boxes, your values for <i>δ</i> and/or <i>ε</i> are probably wrong.")		 {{{#!sagecell
pretty_print(html("<h2>Limits: <i>ε-δ</i></h2>"))
pretty_print(html("This allows you to estimate which values of <i>δ</i> guarantee that <i>f</i> is within <i>ε</i> units of a limit."))
pretty_print(html("<ul><li>Modify the value of <i>f</i> to choose a function.</li>"))
pretty_print(html("<li>Modify the value of <i>a</i> to change the <i>x</i>-value where the limit is being estimated.</li>"))
pretty_print(html("<li>Modify the value of <i>L</i> to change your guess of the limit.</li>"))
pretty_print(html("<li>Modify the values of <i>δ</i> and <i>ε</i> to modify the rectangle.</li></ul>"))
pretty_print(html("If the blue curve passes through the pink boxes, your values for <i>δ</i> and/or <i>ε</i> are probably wrong."))
Line 563: Line 579:
{{{ {{{#!sagecell
Line 567: Line 583:
    html('<h3>A graphical illustration of $\lim_{x -> 0} \sin(x)/x =1$</h3>')
    html('Below is the unit circle, so the length of the <font color=red>red line</font> is |sin(x)|')
    html('and the length of the <font color=blue>blue line</font> is |tan(x)| where x is the length of the arc.') 
    html('From the picture, we see that |sin(x)| $\le$ |x| $\le$ |tan(x)|.')
    html('It follows easily from this that cos(x) $\le$ sin(x)/x $\le$ 1 when x is near 0.')
    html('As $\lim_{x ->0} \cos(x) =1$, we conclude that $\lim_{x -> 0} \sin(x)/x =1$.')		     pretty_print(html('<h3>A graphical illustration of $\lim_{x -> 0} \sin(x)/x =1$</h3>'))
    pretty_print(html('Below is the unit circle, so the length of the <font color=red>red line</font> is |sin(x)|'))
    pretty_print(html('and the length of the <font color=blue>blue line</font> is |tan(x)| where x is the length of the arc.'))
    pretty_print(html('From the picture, we see that |sin(x)| $\le$ |x| $\le$ |tan(x)|.'))
    pretty_print(html('It follows easily from this that cos(x) $\le$ sin(x)/x $\le$ 1 when x is near 0.'))
    pretty_print(html('As $\lim_{x ->0} \cos(x) =1$, we conclude that $\lim_{x -> 0} \sin(x)/x =1$.'))
Line 589: Line 605:
{{{ {{{#!sagecell
Line 604: Line 620:
{{{ {{{#!sagecell
Line 623: Line 639:
sin,cos = math.sin,math.cos
html("<h1>The midpoint rule for a function of two variables</h1>")
pretty_print(html("<h1>The midpoint rule for a function of two variables</h1>"))
Line 639: Line 655:
    html("$$\int_{"+str(R16(y_start))+"}^{"+str(R16(y_end))+"} "+ "\int_{"+str(R16(x_start))+"}^{"+str(R16(x_end))+"} "+func+"\ dx \ dy$$")
    html('<p style="text-align: center;">Numerical approximation: ' + str(num_approx)+'</p>')		     pretty_print(html("$$\int_{"+str(R16(y_start))+"}^{"+str(R16(y_end))+"} "+ "\int_{"+str(R16(x_start))+"}^{"+str(R16(x_end))+"} "+func+"\ dx \ dy$$"))
    pretty_print(html('<p style="text-align: center;">Numerical approximation: ' + str(num_approx)+'</p>'))
Line 650: Line 666:
{{{
from scipy.special.orthogonal import p_roots		 {{{#!sagecell
import scipy
import numpy
from scipy.special.orthogonal import p_roots, t_roots, u_roots
Line 660: Line 678:
            'Chebyshev': {'w': 1/sqrt(1-x**2), 'xmin': -1, 'xmax': 1, 'func': t_roots},
                'Chebyshev2': {'w': sqrt(1-x**2), 'xmin': -1, 'xmax': 1, 'func': u_roots},
                'Trapezoid': {'w': 1, 'xmin': -1, 'xmax': 1, 'func': lambda n: (linspace(-1r,1,n), numpy.array([1.0r]+[2.0r]*(n-2)+[1.0r])*1.0r/n)},
                'Simpson': {'w': 1, 'xmin': -1, 'xmax': 1, 'func': lambda n: (linspace(-1r,1,n), numpy.array([1.0r]+[4.0r,2.0r]*int((n-3.0r)/2.0r)+[4.0r,1.0r])*2.0r/(3.0r*n))}}		      'Chebyshev': {'w': 1/sqrt(1-x**2), 'xmin': -1, 'xmax': 1, 'func': t_roots},
     'Chebyshev2': {'w': sqrt(1-x**2), 'xmin': -1, 'xmax': 1, 'func': u_roots},
     'Trapezoid': {'w': 1, 'xmin': -1, 'xmax': 1,          'func': lambda n: (linspace(-1r,1,n), numpy.array([1.0r]+[2.0r]*(n-2)+[1.0r])*1.0r/n)},
     'Simpson': {'w': 1, 'xmin': -1, 'xmax': 1,          'func': lambda n: (linspace(-1r,1,n),
            numpy.array([1.0r]+[4.0r,2.0r]*int((n-3.0r)/2.0r)+[4.0r,1.0r])*2.0r/(3.0r*n))}}
Line 667: Line 688:
    return polygon([(center-width2,0),(center+width2,0),(center+width2,height),(center-width2,height)],**kwds)     return polygon([(center-width2,0),
        (center+width2,0),(center+width2,height),(center-width2,height)],**kwds)
Line 671: Line 693:
def weights(n=slider(1,30,1,default=10),f=input_box(default=3*x+cos(10*x)),show_method=["Legendre", "Chebyshev", "Chebyshev2", "Trapezoid","Simpson"]): def weights(n=slider(1,30,1,default=10),f=input_box(default=3*x+cos(10*x),type=SR),
    show_method=["Legendre", "Chebyshev", "Chebyshev2", "Trapezoid","Simpson"]):
Line 680: Line 703:
    scaled_ff = fast_float(scaled_func)     scaled_ff = fast_float(scaled_func, 'x')
Line 688: Line 711:
    stems = sum(line([(x,0),(x,scaled_ff(x))],rgbcolor=(1-y,1-y,1-y),thickness=2,markersize=6,alpha=y) for x,y in coords_scaled)
    points = sum([point([(x,0),(x,scaled_ff(x))],rgbcolor='black',pointsize=30) for x,_ in coords])		     stems = sum(line([(x,0),(x,scaled_ff(x))],rgbcolor=(1-y,1-y,1-y),
        thickness=2,markersize=6,alpha=y) for x,y in coords_scaled)
    points = sum([point([(x,0),
        (x,scaled_ff(x))],rgbcolor='black',pointsize=30) for x,_ in coords])
Line 694: Line 719:
    show(graph,xmin=plot_min,xmax=plot_max)     show(graph,xmin=plot_min,xmax=plot_max,aspect_ratio="auto")
Line 702: Line 727:
    html("$$\sum_{i=1}^{i=%s}w_i\left(%s\\right)= %s\\approx %s =\int_{-1}^{1}%s \,dx$$"%(n,latex(f.subs(x="x_i")), approximation, integral, latex(scaled_func)))     pretty_print(html("$$\sum_{i=1}^{i=%s}w_i\left(%s\\right)= %s\\approx %s =\int_{-1}^{1}%s \,dx$$"%(n,
        latex(f), approximation, integral, latex(scaled_func))))
Line 715: Line 741:
{{{ {{{#!sagecell
Line 746: Line 772:
velocity = derivative( position(t) )
acceleration = derivative(velocity(t))		 velocity = derivative(position(t), t)
acceleration = derivative(velocity(t), t)
Line 749: Line 775:
speed_deriv = derivative(speed) speed_deriv = derivative(speed, t)
Line 751: Line 777:
dT = derivative(tangent(t)) dT = derivative(tangent(t), t)
Line 822: Line 848:
== Vector Calculus, 3-D Motion == == Vector Calculus, 3-D Motion (FIXME) ==
Line 827: Line 853:
{{{ {{{#!sagecell
Line 864: Line 890:
velocity = derivative( position(t) )
acceleration = derivative(velocity(t))		 velocity = derivative( position(t), t)
acceleration = derivative(velocity(t), t)
Line 867: Line 893:
speed_deriv = derivative(speed) speed_deriv = derivative(speed, t)
Line 869: Line 895:
dT = derivative(tangent(t)) dT = derivative(tangent(t), t)
Line 872: Line 898:
## dB = derivative(binormal(t)) ## dB = derivative(binormal(t), t)
Line 953: Line 979:
http://www.sagenb.org/home/pub/2828/

{{{		 http://sagenb.mc.edu/home/pub/97/

{{{#!sagecell
Line 963: Line 989:
## An updated version of this worksheet may be available at http://sagenb.mc.edu
Line 968: Line 993:
var('x,y,z')
Rmin=1/10
Line 970: Line 996:
@interact
def _(f=input_box(default=(x^3-y^3)/(x^2+y^2)),R=slider(0.1/10,Rmax,1/10,2),x0=(0),y0=(0)):		 @interact(layout=dict(top=[['f'],['x0'],['y0']],
bottom=[['in_3d','curves','R','graphjmol']]))
def _(f=input_box((x^2-y^2)/(x^2+y^2),width=30,label='$f(x)$'),
        R=slider(Rmin,Rmax,1/10,Rmax,label=',   $R$'),
        x0=input_box(0,width=10,label='$x_0$'),
        y0=input_box(0,width=10,label='$y_0$'),
        curves=checkbox(default=false,label='Show curves'),
        in_3d=checkbox(default=false,label='3D'),
        graphjmol=checkbox(default=true,label='Interactive graph')):
    if graphjmol:
        view_method = 'jmol'
    else:
        view_method = 'tachyon'
Line 979: Line 1016:
    
Line 981: Line 1018:
    limit = plot3d(g,(t,0,2*pi),(r,1/100,R),transformation=cylinder,rgbcolor=(0,1,0))     collapsing_surface = plot3d(g,(t,0,2*pi),(r,1/100,R),transformation=cylinder,rgbcolor=(0,1,0))
Line 983: Line 1020:
    show(surface+limit)
    print html('Enter $(x_0 ,y_0 )$ above and see what happens as R approaches zero.')
    print html('The surface has a limit as $(x,y)$ approaches ('+str(x0)+','+str(y0)+') if the green region collapses to a point')		     G = surface+collapsing_surface
    pretty_print(html('Enter $(x_0 ,y_0 )$ above and see what happens as $ R \\rightarrow 0 $.'))
    pretty_print(html('The surface has a limit as $(x,y) \\rightarrow $ ('+str(x0)+','+str(y0)+') if the green region collapses to a point.'))

# If checked, add a couple of curves on the surface corresponding to limit as x->x0 for y=x^(3/5),
# and as y->y0 for x=y^(3/5). Should make this more robust but perhaps using
# these relatively obtuse curves could eliminate problems.

    if curves:
        curve_x = parametric_plot3d([x0-t,y0-t^(3/5),f(x=x0-t,y=y0-t^(3/5))],(t,Rmin,Rmax),color='red',thickness=10)
        curve_y = parametric_plot3d([x0+t^(3/5),y0+t,f(x=x0+t^(3/5),y=y0+t)],(t,Rmin,Rmax),color='red',thickness=10)
        R2 = Rmin/4
        G += arrow((x0-Rmin,y0-Rmin^(3/5),f(x=x0-Rmin,y=y0-Rmin^(3/5))),(x0-R2,y0-R2^(3/5),f(x=x0-R2,y=y0-R2^(3/5))),size=30 )
        G += arrow((x0+Rmin^(3/5),y0+Rmin,f(x=x0+Rmin^(3/5),y=y0+Rmin)),(x0+R2^(3/5),y0+R2,f(x=x0+R2^(3/5),y=y0+R2)),size=30 )

        limit_x = limit(f(x=x0-t,y=y0-t^(3/5)),t=0)
        limit_y = limit(f(x=x0+t^(3/5),y=y0+t),t=0)
        text_x = text3d(limit_x,(x0,y0,limit_x))
        text_y = text3d(limit_y,(x0,y0,limit_y))
        G += curve_x+curve_y+text_x+text_y
 
    
        pretty_print(html('The red curves represent a couple of trajectories on the surface. If they do not meet, then'))
        pretty_print(html('there is also no limit. (If computer hangs up, likely the computer can not do these limits.)'))
        pretty_print(html('\n<center><font color="red">$\lim_{(x,?)\\rightarrow(x_0,y_0)} f(x,y) =%s$</font>'%str(limit_x)+' and <font color="red">$\lim_{(?,y)\\rightarrow(x_0,y_0)} f(x,y) =%s$</font></center>'%str(limit_y)))
        
    if in_3d:
        show(G,stereo="redcyan",viewer=view_method)
    else:
        show(G,perspective_depth=true,viewer=view_method)
Line 990: Line 1054:
{{{ {{{#!sagecell
Line 1007: Line 1072:
Rmax=2
@interact
def _(f=input_box(default=(x^3-y^3)/(x^2+y^2)),
      N=slider(5,100,1,10,label='Number of Contours'),
      x0=(0),y0=(0)):

    print html('Enter $(x_0 ,y_0 )$ above and see what happens as the number of contour levels increases.')
    print html('A surface will have a limit in the center of this graph provided there is not a sudden change in color there.') 		var('x,y,z,u')
@interact(layout=dict(top=[['f'],['x0'],['y0']],
bottom=[['N'],['R']]))
def _(f=input_box(default=(x*y^2)/(x^2+y^4),width=30,label='$f(x)$'),
        N=slider(5,100,1,10,label='Number of Contours'),
        R=slider(0.1,1,0.01,1,label='Radius of circular neighborhood'),
        x0=input_box(0,width=10,label='$x_0$'),
        y0=input_box(0,width=10,label='$y_0$')):

    pretty_print(html('Enter $(x_0 ,y_0 )$ above and see what happens as the number of contour levels $\\rightarrow \infty $.'))
    pretty_print(html('A surface will have a limit in the center of this graph provided there is not a sudden change in color there.'))

# Need to make certain the min and max contour lines are not huge due to asymptotes. If so, clip and start contours at some reasonable
# values so that there are a nice collection of contours to show around the desired point.
Line 1017: Line 1088:
    surface += parametric_plot([R*cos(u),R*sin(u)],[0,2*pi],color='black')
# Nice to use if f=x*y^2/(x^2 + y^4)
# var('u')
# surface += parametric_plot([u^2,u],[u,-1,1],color='black')
Line 1018: Line 1093:
    show(limit_point+surface)}}} # show(limit_point+surface)
    pretty_print(table([[surface],['hi']]))
}}}
Line 1027: Line 1104:
{{{ {{{#!sagecell
Line 1080: Line 1157:
{{{
%hide
%auto		 {{{#!sagecell
Line 1097: Line 1172:
 html(r'Function $ f(x,y)=%s$ '%latex(f(x,y)))  pretty_print(html(r'Function $ f(x,y)=%s$ '%latex(f(x,y))))
Line 1111: Line 1186:
              html(r'<tr><td>$\quad f(%s,%s)\quad $</td><td>$\quad %s$</td>\
              </tr>'%(latex(x0),latex(y0),z0.n()))		               pretty_print(html(r'<tr><td>$\quad f(%s,%s)\quad $</td><td>$\quad %s$</td>\
              </tr>'%(latex(x0),latex(y0),z0.n())))
Line 1141: Line 1216:
{{{ {{{#!sagecell
Line 1145: Line 1220:
html('Points x0 and y0 are values where the exact value of the function \ pretty_print(html('Points x0 and y0 are values where the exact value of the function \
Line 1147: Line 1222:
and approximation by differential at shifted point are compared.') and approximation by differential at shifted point are compared.'))
Line 1165: Line 1240:
  html(r'Function $ f(x,y)=%s \approx %s $ '%(latex(f(x,y)),latex(tangent(x,y))))
  html(r' $f %s = %s$'%(latex((x0,y0)),latex(exact_value_ori)))
  html(r'Shifted point $%s$'%latex(((x0+deltax),(y0+deltay))))
  html(r'Value of the function in shifted point is $%s$'%f(x0+deltax,y0+deltay))
  html(r'Value on the tangent plane in shifted point is $%s$'%latex(approx_value))
  html(r'Error is $%s$'%latex(abs_error)) 		   pretty_print(html(r'Function $ f(x,y)=%s \approx %s $ '%(latex(f(x,y)),latex(tangent(x,y)))))
  pretty_print(html(r' $f %s = %s$'%(latex((x0,y0)),latex(exact_value_ori))))
  pretty_print(html(r'Shifted point $%s$'%latex(((x0+deltax),(y0+deltay)))))
  pretty_print(html(r'Value of the function in shifted point is $%s$'%f(x0+deltax,y0+deltay)))
  pretty_print(html(r'Value on the tangent plane in shifted point is $%s$'%latex(approx_value)))
  pretty_print(html(r'Error is $%s$'%latex(abs_error)))
Line 1179: Line 1254:
{{{ {{{#!sagecell
Line 1207: Line 1282:
    html('$F(x,y) = e^{-(x^2+y^2)/2} \\cos(y) \\sin(x^2+y^2)$')     pretty_print(html('$F(x,y) = e^{-(x^2+y^2)/2} \\cos(y) \\sin(x^2+y^2)$'))
Line 1217: Line 1292:
http://www.sagenb.org/home/pub/2829/

{{{		 https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2829.sagews

{{{#!sagecell
Line 1320: Line 1395:
== Lateral Surface Area == == Lateral Surface Area (FIXME in Jupyter) ==
Line 1324: Line 1399:
http://www.sagenb.org/home/pub/2826/

{{{		 http://sagenb.mc.edu/home/pub/89/

{{{#!sagecell
Line 1331: Line 1406:
##
Line 1333: Line 1409:
@interact
def _(f=input_box(default=6-4*x^2-y^2*2/5,label='$f(x,y) = $'),
        g=input_box(default=-2+sin(x)+sin(y),label='$g(x,y) = $'),
        u=input_box(default=cos(t),label='$u(t) = $'),
        v=input_box(default=2*sin(t),label='$v(t) = $'),
        a=input_box(default=0,label='$a = $'),
        b=input_box(default=3*pi/2,label='$b = $'),		 @interact(layout=dict(top=[['f','u'],['g','v']],
left=[['a'],['b'],['in_3d'],['smoother']],
bottom=[['xx','yy']]))
def _(f=input_box(default=6-4*x^2-y^2*2/5,label='Top = $f(x,y) = $',width=30),
        g=input_box(default=-2+sin(x)+sin(y),label='Bottom = $g(x,y) = $',width=30),
        u=input_box(default=cos(t),label='   $ x = u(t) = $',width=20),
        v=input_box(default=2*sin(t),label='   $ y = v(t) = $',width=20),
        a=input_box(default=0,label='$a = $',width=10),
        b=input_box(default=3*pi/2,label='$b = $',width=10),
Line 1342: Line 1420:
        smoother=checkbox(default=false)):         in_3d = checkbox(default=true,label='3D'),
 smoother=checkbox(default=false),
        auto_update=true):
Line 1344: Line 1424:
    ds = sqrt(derivative(u(t),t)^2+derivative(v(t),t)^2)     ds = sqrt(derivative(u,t)^2+derivative(v,t)^2)
Line 1348: Line 1428:
    A = (f(x=u(t),y=v(t))-g(x=u(t),y=v(t)))*ds.simplify_trig().simplify()     A = (f(x=u,y=v)-g(x=u,y=v))*ds.simplify_trig().simplify()
Line 1353: Line 1433:
    line_integral = integral(A,t,a,b) # If you want Sage to try, uncomment the lines below.

# line_integral = integrate(A,t,a,b)
# html(r'<align=center size=+1>Lateral Surface Area = $ %s $ </font>'%latex(line_integral))
Line 1355: Line 1439:
       
    html(r'<h4 align=center>Lateral Surface Area = $ %s $ </h4>'%latex(line_integral))

    html(r'<h4 align=center>Lateral Surface $ \approx $ %s</h2>'%str(line_integral_approx))
    pretty_print(html(r'<font align=center size=+1>Lateral Surface $ \approx $ %s</font>'%str(line_integral_approx)))
Line 1365: Line 1447:
    G += parametric_plot3d([u,v,g(x=u(t),y=v(t))],(t,a,b),thickness=2,color='red')
    G += parametric_plot3d([u,v,f(x=u(t),y=v(t))],(t,a,b),thickness=2,color='red')		     G += parametric_plot3d([u,v,g(x=u,y=v)],(t,a,b),thickness=2,color='red')
    G += parametric_plot3d([u,v,f(x=u,y=v)],(t,a,b),thickness=2,color='red')
Line 1375: Line 1457:
        G += parametric_plot3d([u(w),v(w),s*f(x=u(w),y=v(w))+(1-s)*g(x=u(w),y=v(w))],(s,0,1),thickness=lat_thick,color='yellow',opacity=0.9)
    show(G,spin=true)		         G += parametric_plot3d([u(t=w),v(t=w),s*f(x=u(t=w),y=v(t=w))+(1-s)*g(x=u(t=w),y=v(t=w))],(s,0,1),thickness=lat_thick,color='yellow',opacity=0.9)
             if in_3d:
        show(G,stereo='redcyan',spin=true)
    else:
        show(G,perspective_depth=true,spin=true)
Line 1381: Line 1467:
== Parametric surface example == == Parametric surface example (FIXME in Jupyter) ==
Line 1383: Line 1469:
{{{ {{{#!sagecell
Line 1402: Line 1488:
http://www.sagenb.org/home/pub/2827/

{{{		 https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2827-$%20%5Cint_%7BC%7D%20%5Cleft%20%5Clangle%20M,N,P%20%5Cright%20%5Crangle%20dr%20$%20=%20$%20%25s%20$.sagews

{{{#!sagecell
Line 1438: Line 1524:
    html(r'<h2 align=center>$ \int_{C} \left \langle M,N,P \right \rangle dr $ = $ %s $ </h2>'%latex(line_integral))     pretty_print(html(r'<h2 align=center>$ \int_{C} \left \langle M,N,P \right \rangle dr $ = $ %s $ </h2>'%latex(line_integral)))

Sage Interactions - Calculus

goto interact main page

Contents

  1. Sage Interactions - Calculus
    1. Root Finding Using Bisection
    2. Newton's Method
    3. A contour map and 3d plot of two inverse distance functions
    4. A simple tangent line grapher
    5. Numerical integrals with the midpoint rule
    6. Numerical integrals with various rules
    7. Some polar parametric curves
    8. Function tool
    9. Newton-Raphson Root Finding
    10. Coordinate Transformations (FIXME in Jupyter)
    11. Taylor Series
    12. Illustration of the precise definition of a limit
    13. A graphical illustration of sin(x)/x -> 1 as x-> 0
    14. Quadric Surface Plotter
    15. The midpoint rule for numerically integrating a function of two variables
    16. Gaussian (Legendre) quadrature
    17. Vector Calculus, 2-D Motion
    18. Vector Calculus, 3-D Motion (FIXME)
    19. Multivariate Limits by Definition
    20. Directional Derivatives
    21. 3D graph with points and curves
    22. Approximating function in two variables by differential
    23. Taylor approximations in two variables
    24. Volumes over non-rectangular domains
    25. Lateral Surface Area (FIXME in Jupyter)
    26. Parametric surface example (FIXME in Jupyter)
    27. Line Integrals in 3D Vector Field

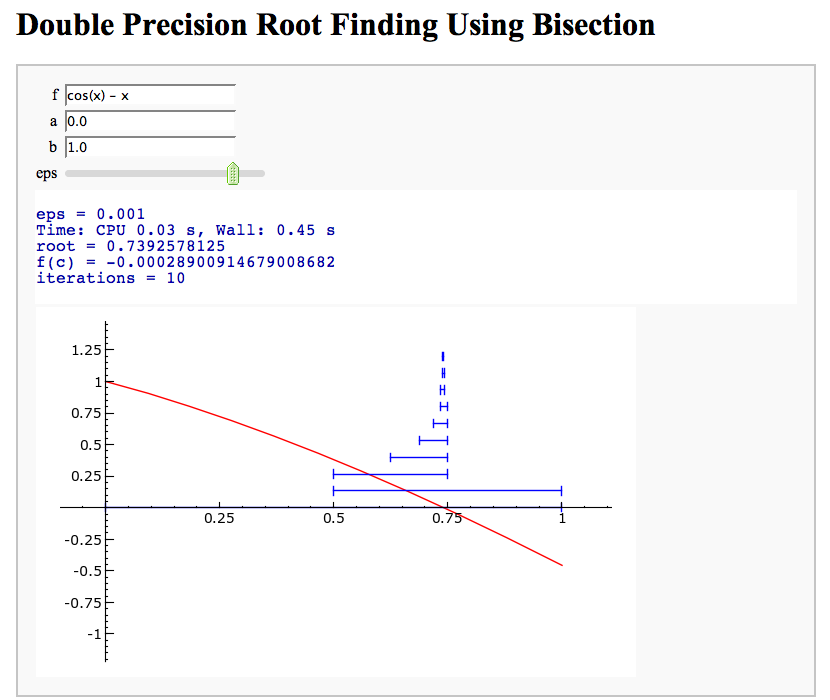
Root Finding Using Bisection

by William Stein

bisect.png

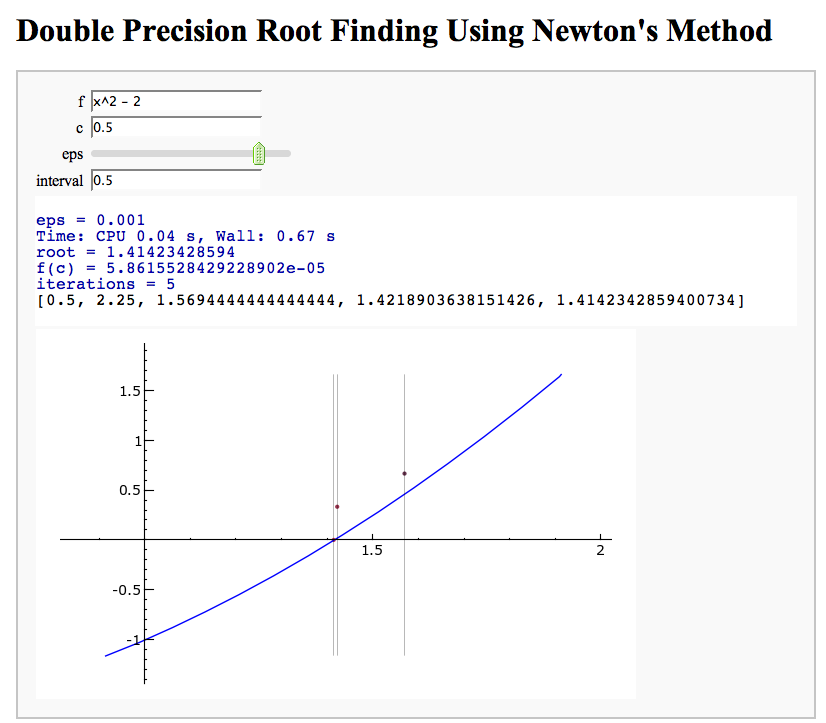
Newton's Method

Note that there is a more complicated Newton's method below.

by William Stein

https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2824-Double%20Precision%20Root%20Finding%20Using%20Newton's%20Method.sagews

newton.png

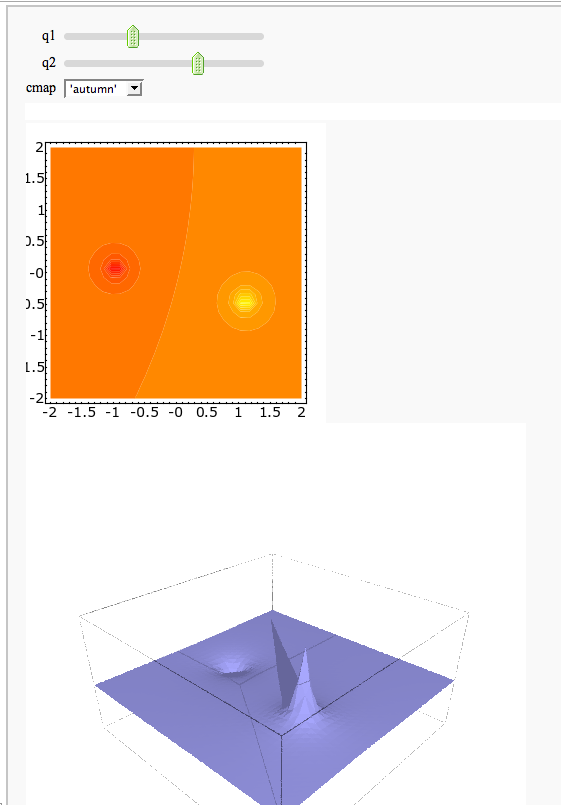
A contour map and 3d plot of two inverse distance functions

by William Stein

https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2823.sagews

mountains.png

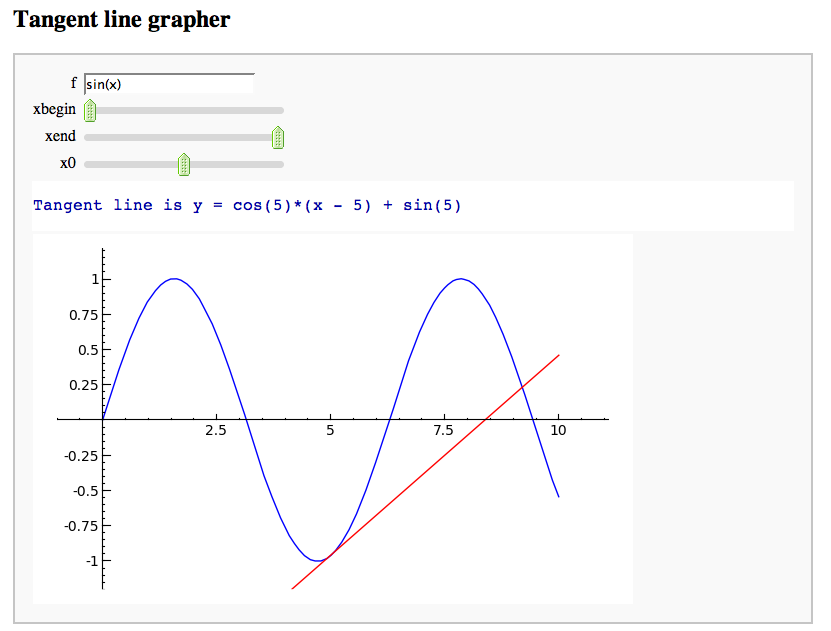
A simple tangent line grapher

by Marshall Hampton

tangents.png

Numerical integrals with the midpoint rule

by Marshall Hampton

num_int.png

Numerical integrals with various rules

by Nick Alexander (based on the work of Marshall Hampton)

num_int2.png

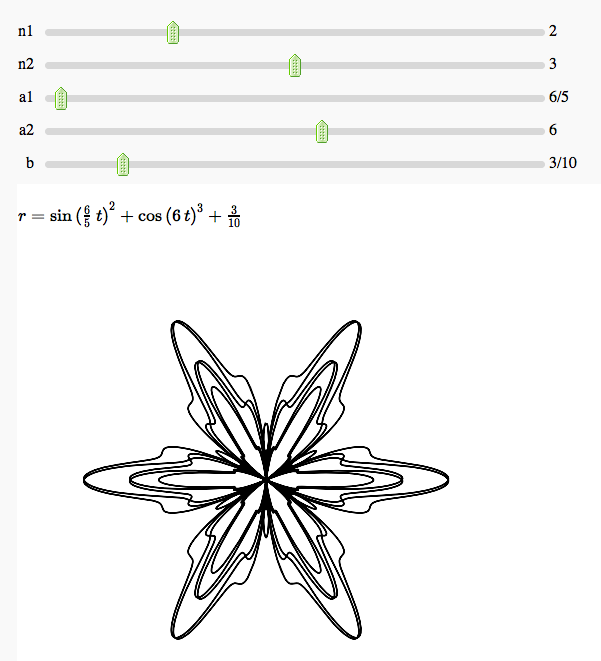
Some polar parametric curves

by Marshall Hampton. This is not very general, but could be modified to show other families of polar curves.

polarcurves1.png

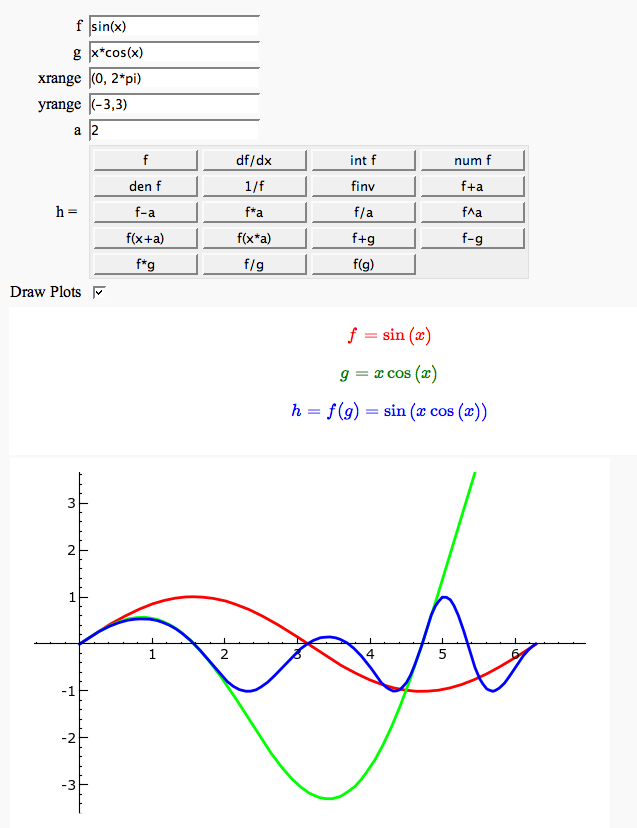
Function tool

Enter symbolic functions f, g, and a, a range, then click the appropriate button to compute and plot some combination of f, g, and a along with f and g. This is inspired by the Matlab funtool GUI.

funtool.png

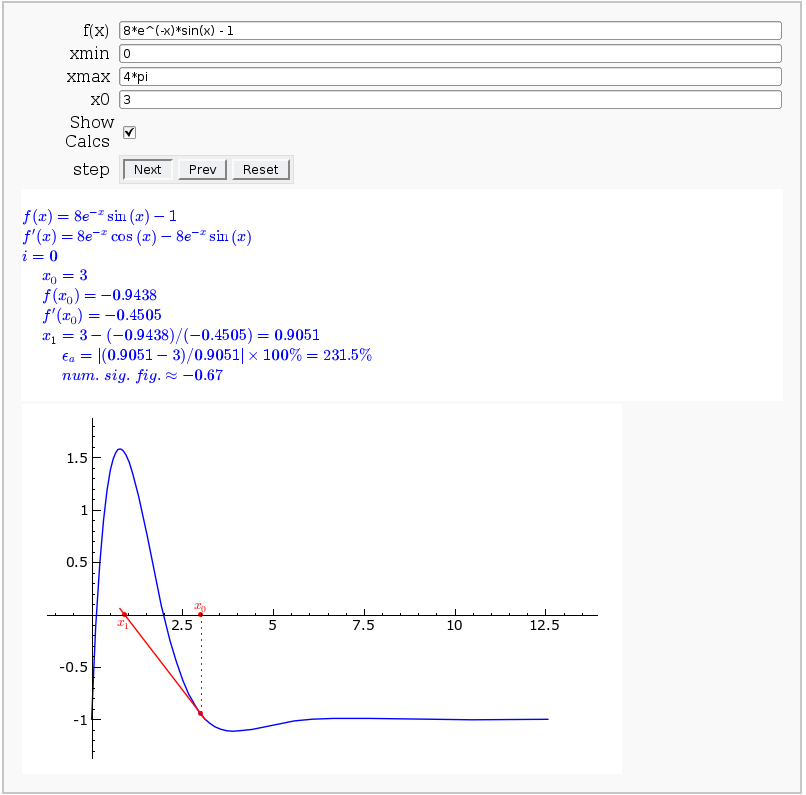
Newton-Raphson Root Finding

by Neal Holtz

This allows user to display the Newton-Raphson procedure one step at a time. It uses the heuristic that, if any of the values of the controls change, then the procedure should be re-started, else it should be continued.

newtraph.png

Coordinate Transformations (FIXME in Jupyter)

by Jason Grout

coordinate-transform-1.png coordinate-transform-2.png

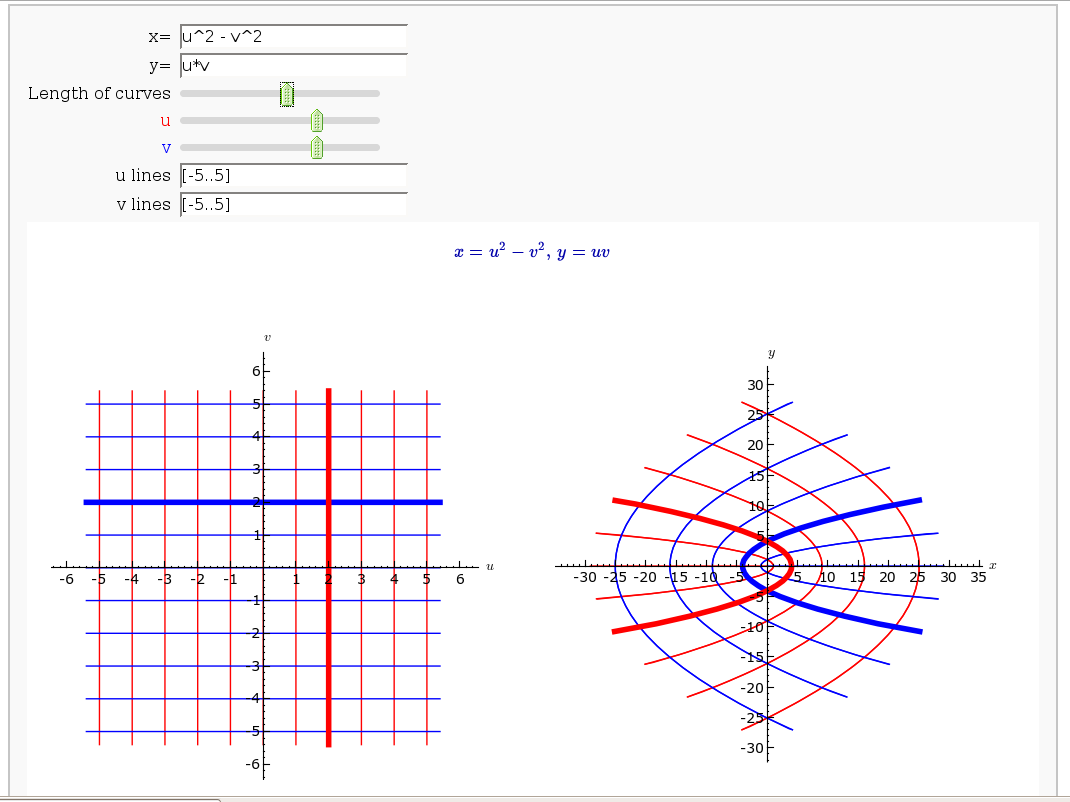
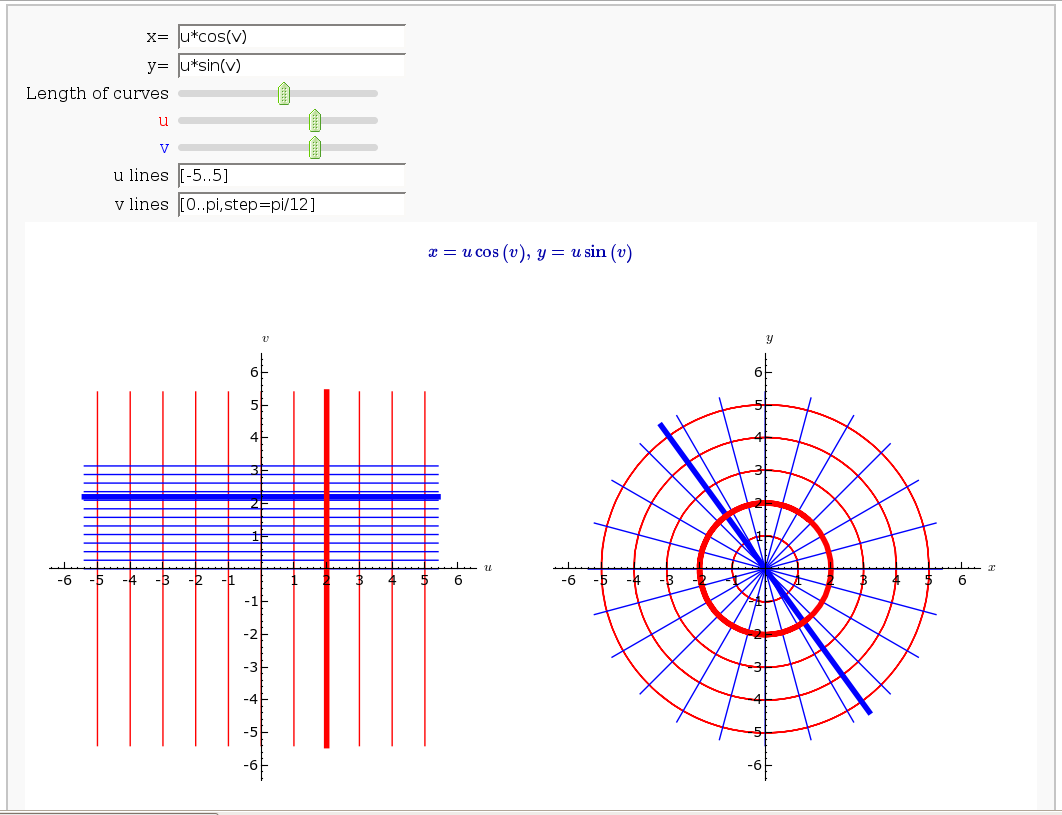
Taylor Series

by Harald Schilly

taylor_series_animated.gif

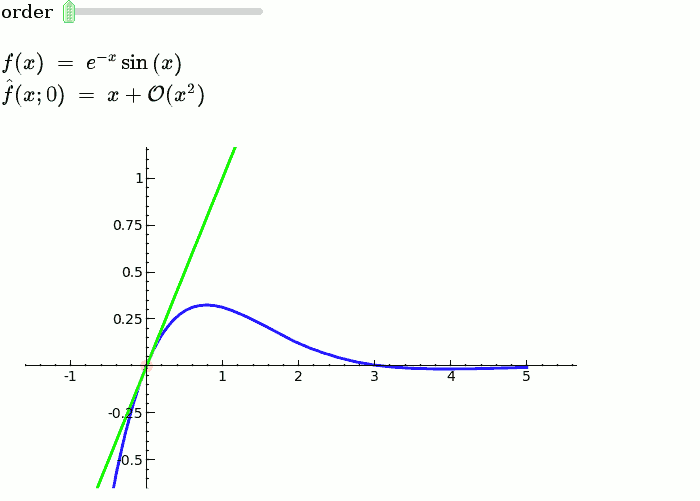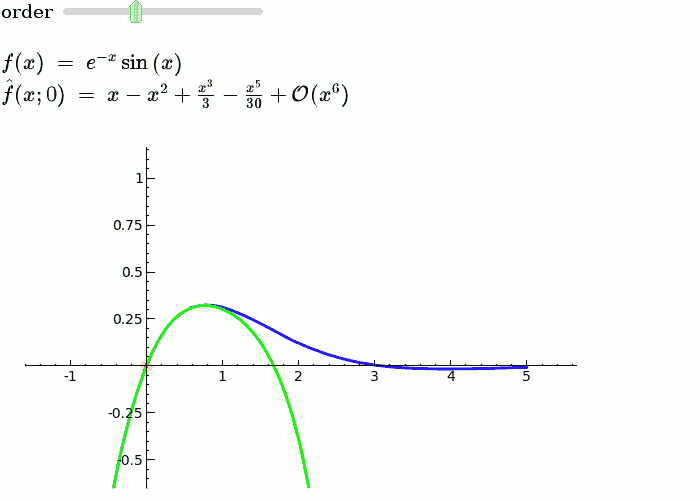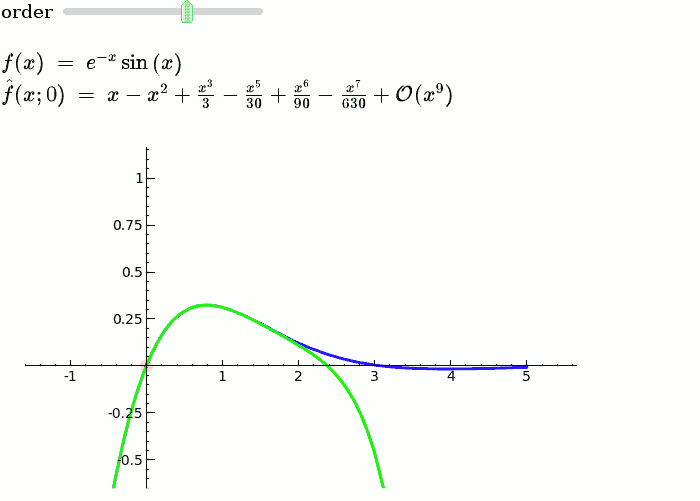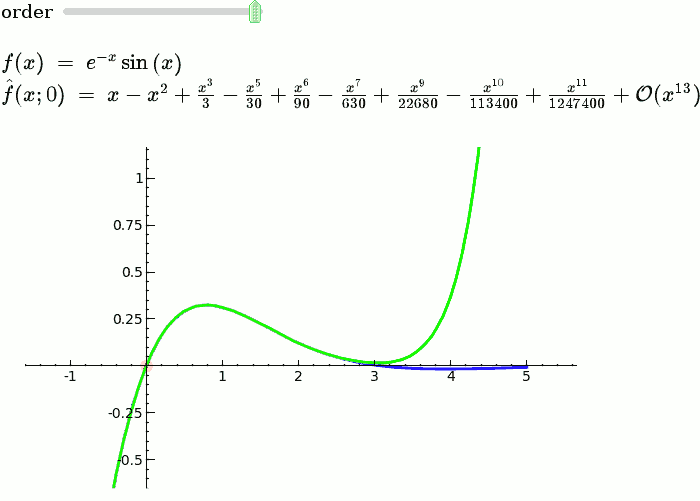
Illustration of the precise definition of a limit

by John Perry

I'll break tradition and put the image first. Apologies if this is Not A Good Thing.

snapshot_epsilon_delta.png

A graphical illustration of sin(x)/x -> 1 as x-> 0

by Wai Yan Pong

sinelimit.png

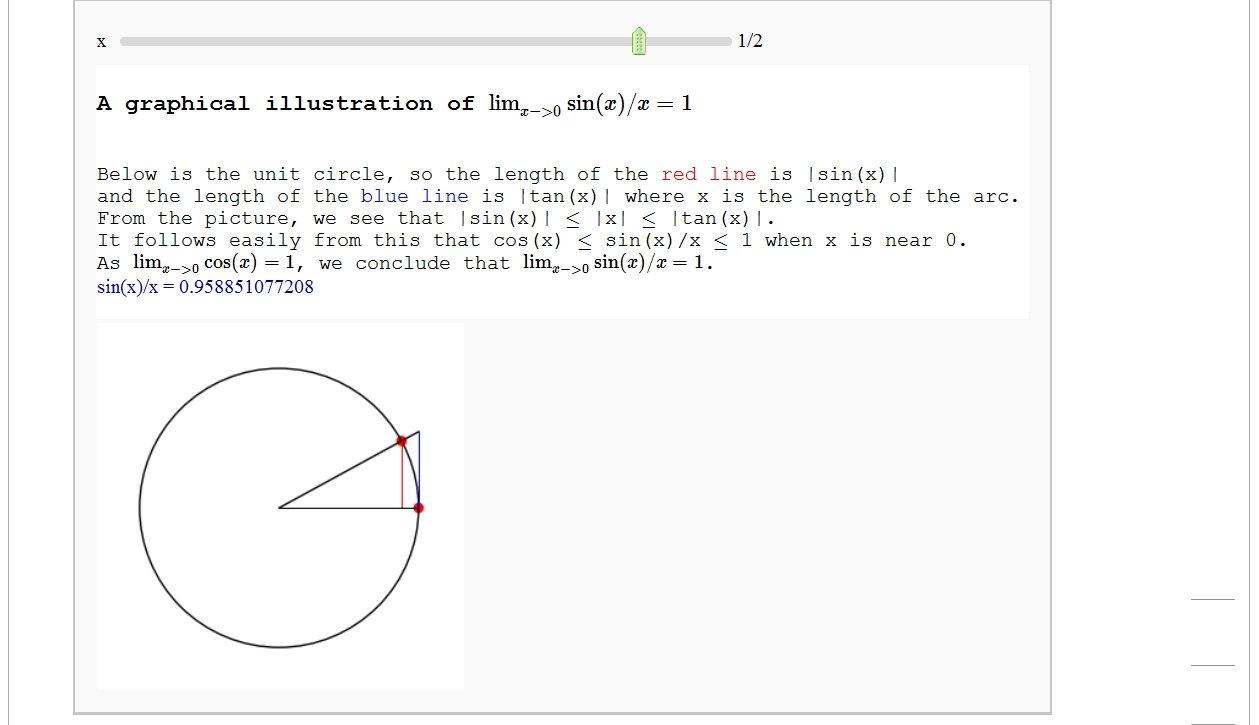
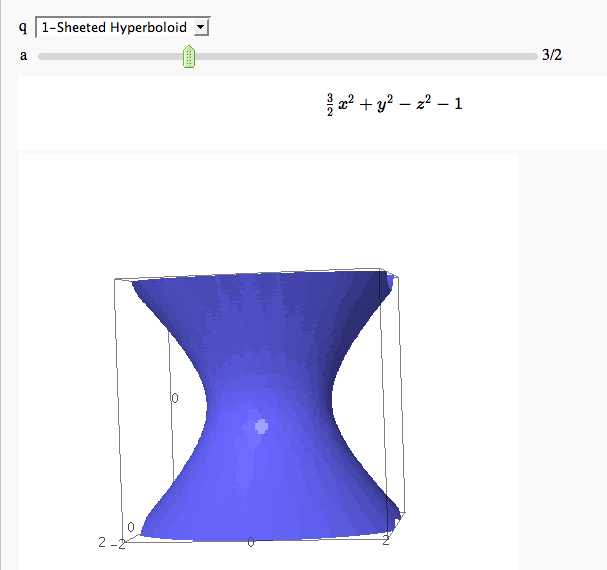
Quadric Surface Plotter

by Marshall Hampton. This is pretty simple, so I encourage people to spruce it up. In particular, it isn't set up to show all possible types of quadrics.

quadrics.png

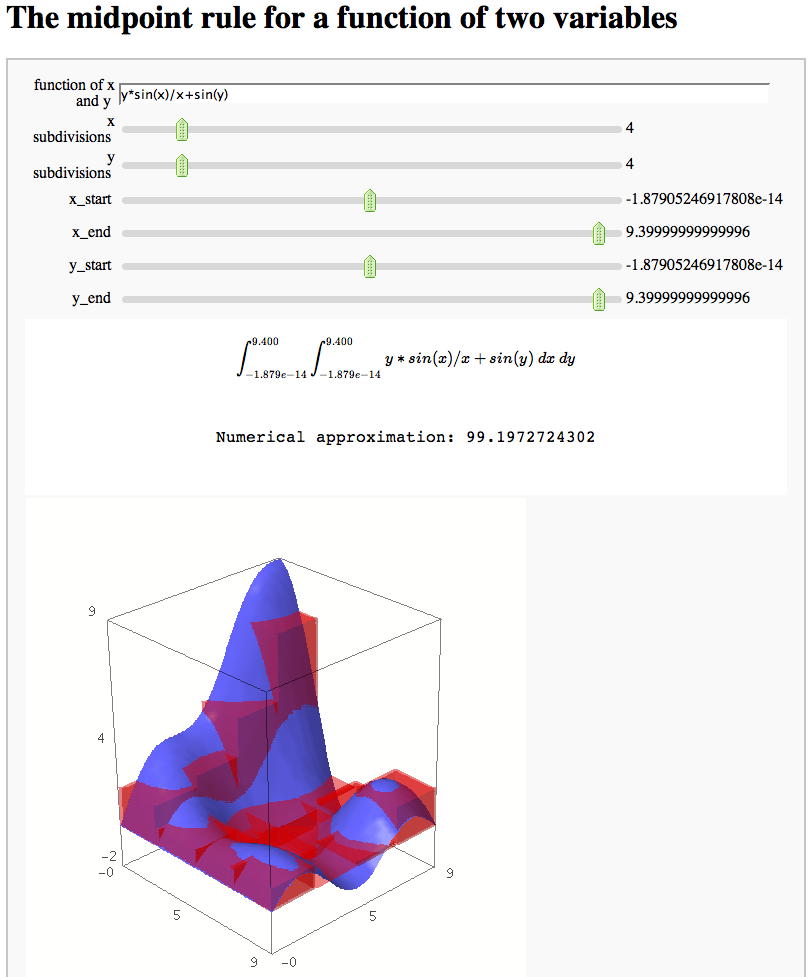
The midpoint rule for numerically integrating a function of two variables

by Marshall Hampton

numint2d.png

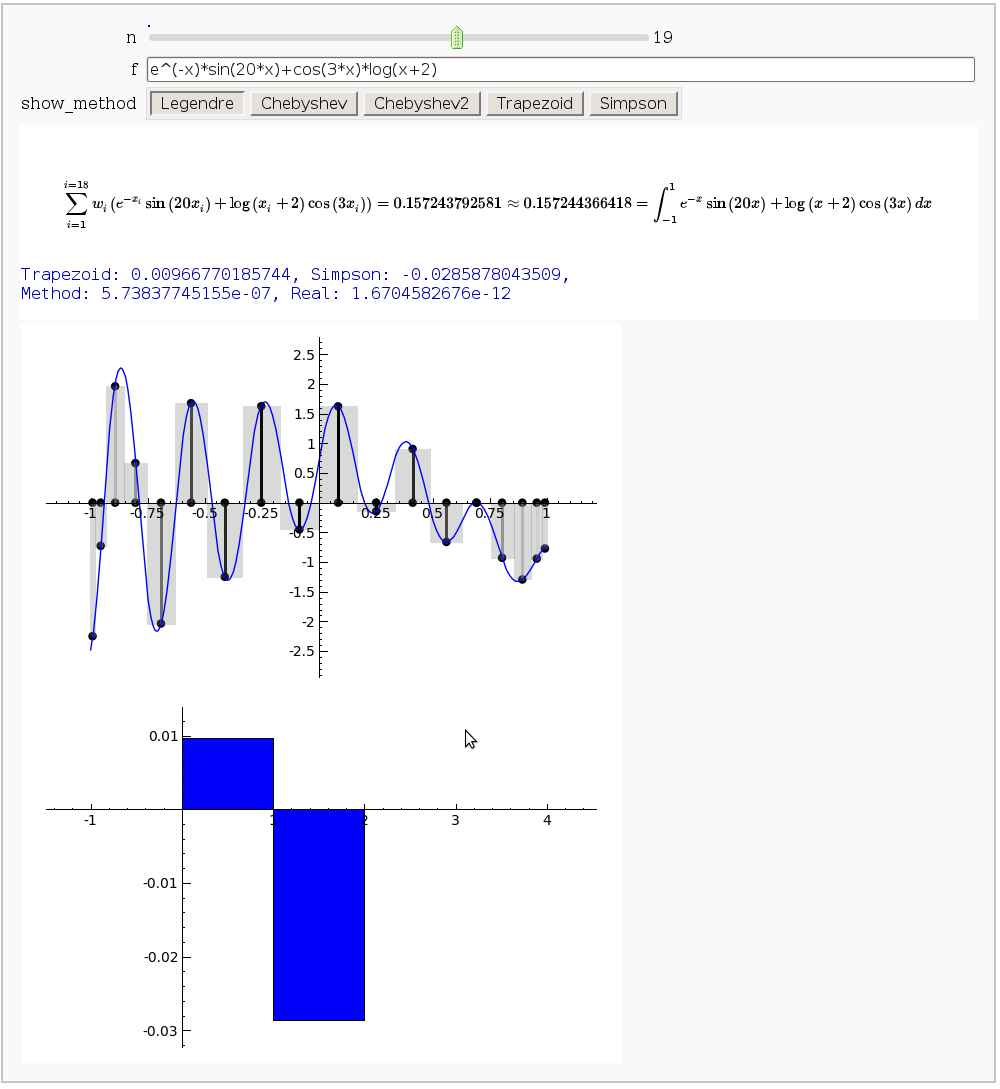
Gaussian (Legendre) quadrature

by Jason Grout

The output shows the points evaluated using Gaussian quadrature (using a weight of 1, so using Legendre polynomials). The vertical bars are shaded to represent the relative weights of the points (darker = more weight). The error in the trapezoid, Simpson, and quadrature methods is both printed out and compared through a bar graph. The "Real" error is the error returned from scipy on the definite integral.

quadrature1.png quadrature2.png

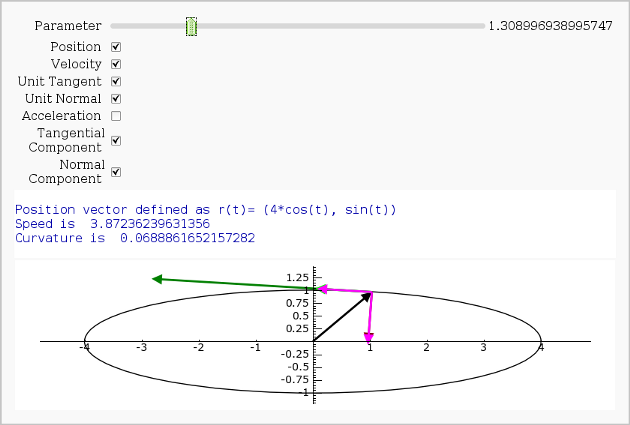
Vector Calculus, 2-D Motion

By Rob Beezer

A fast_float() version is available in a worksheet

motion2d.png

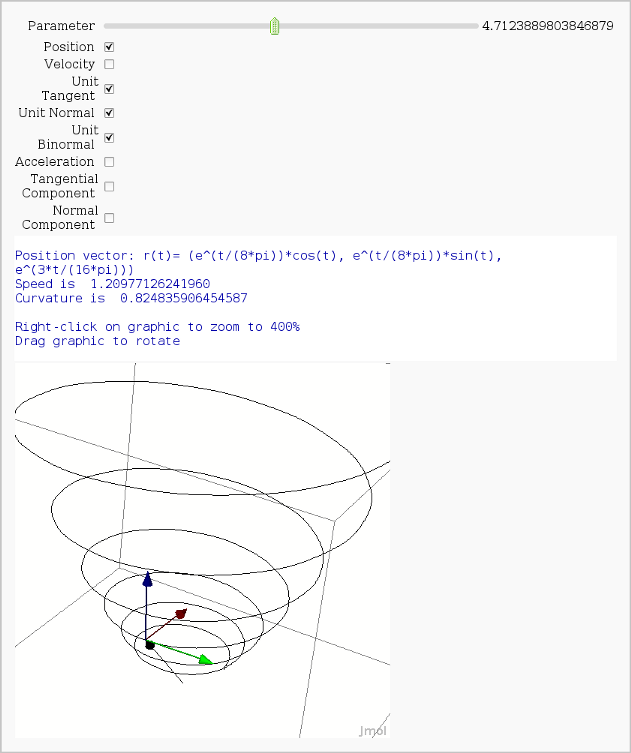
Vector Calculus, 3-D Motion (FIXME)

by Rob Beezer

Available as a worksheet

motion3d.png

Multivariate Limits by Definition

by John Travis

http://sagenb.mc.edu/home/pub/97/

3D_Limit_Defn.png

3D_Limit_Defn_Contours.png

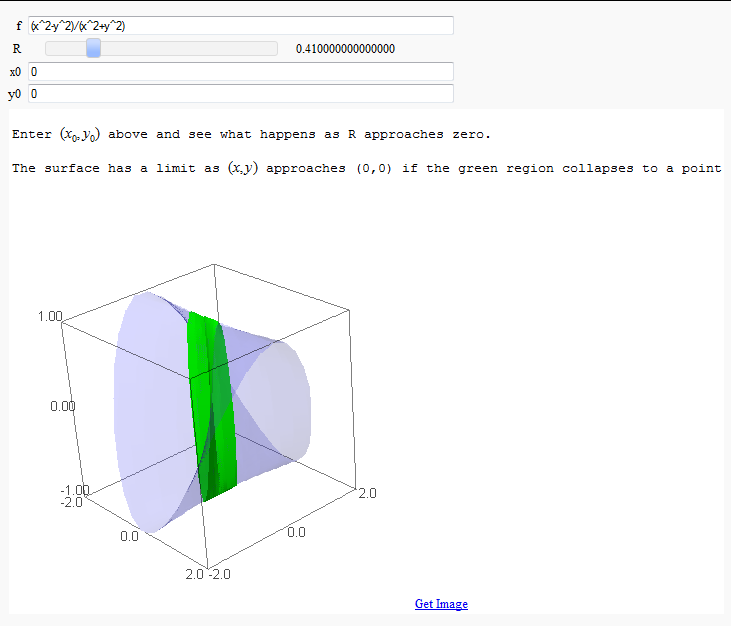
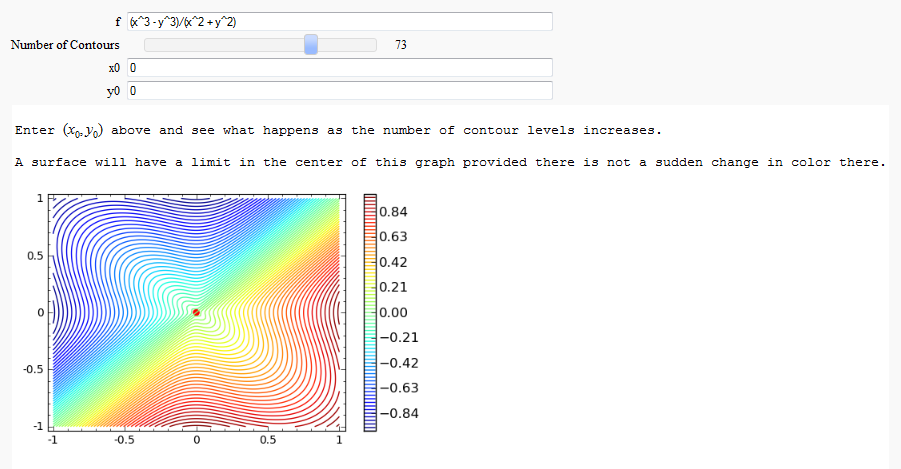
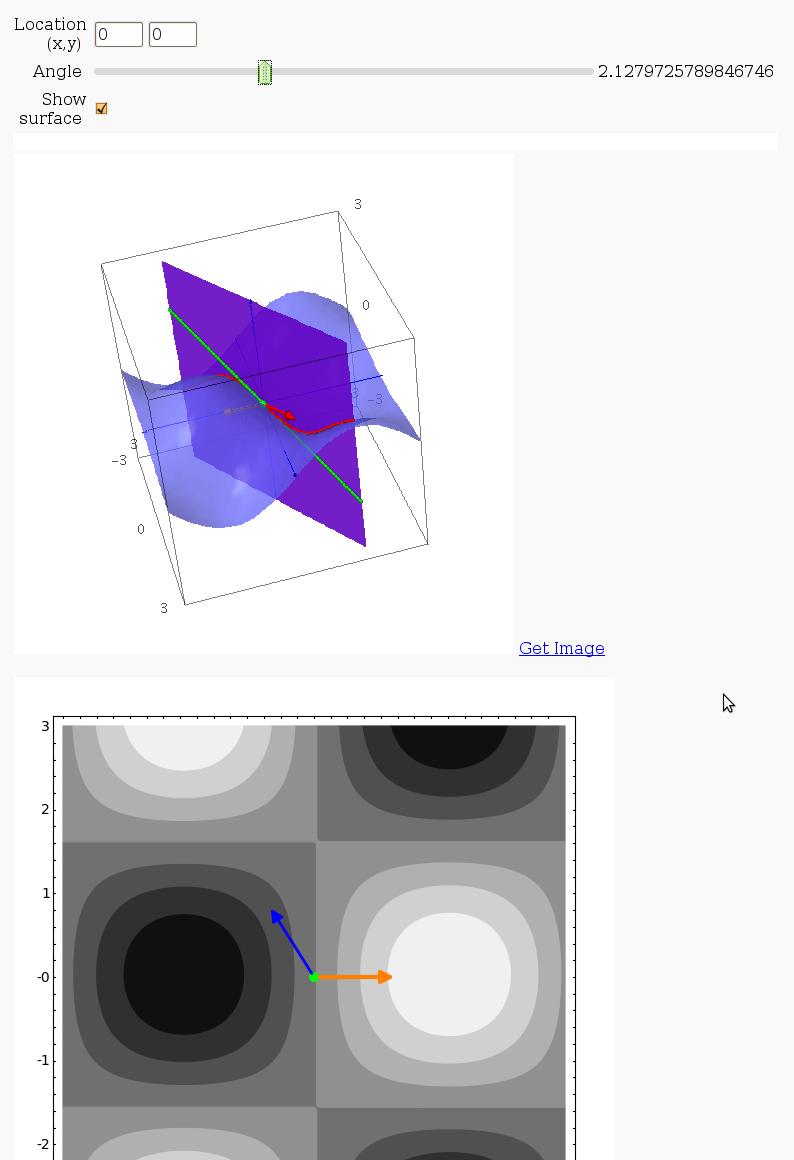
Directional Derivatives

This interact displays graphically a tangent line to a function, illustrating a directional derivative (the slope of the tangent line).

directional derivative.png

3D graph with points and curves

By Robert Marik

This sagelet is handy when showing local, constrained and absolute maxima and minima in two variables. Available as a worksheet

3Dgraph_with_points.png

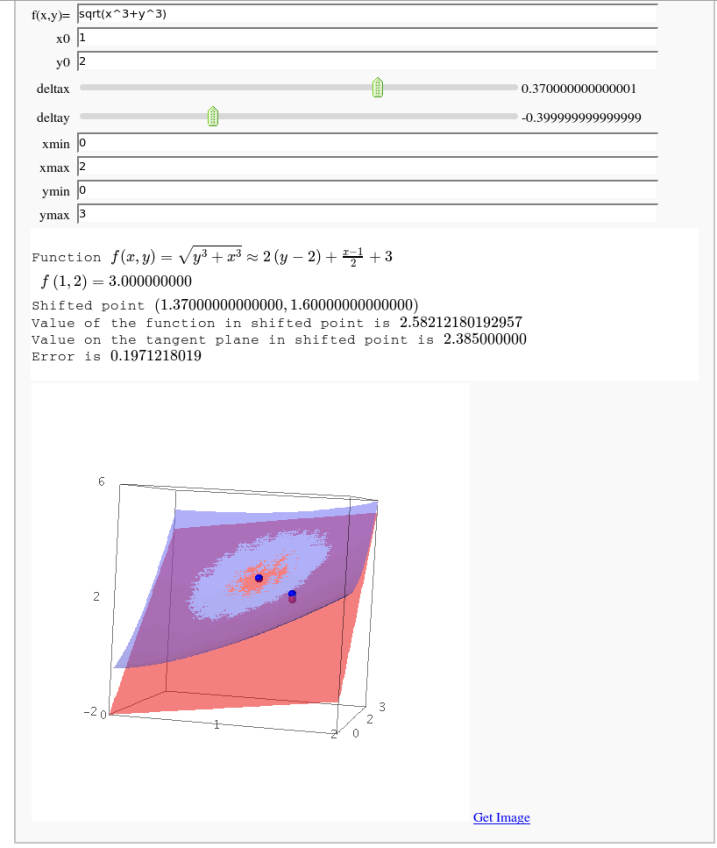
Approximating function in two variables by differential

by Robert Marik

3D_differential.png

Taylor approximations in two variables

by John Palmieri

This displays the nth order Taylor approximation, for n from 1 to 10, of the function sin(x2 + y2) cos(y) exp(-(x2+y2)/2).

taylor-3d.png

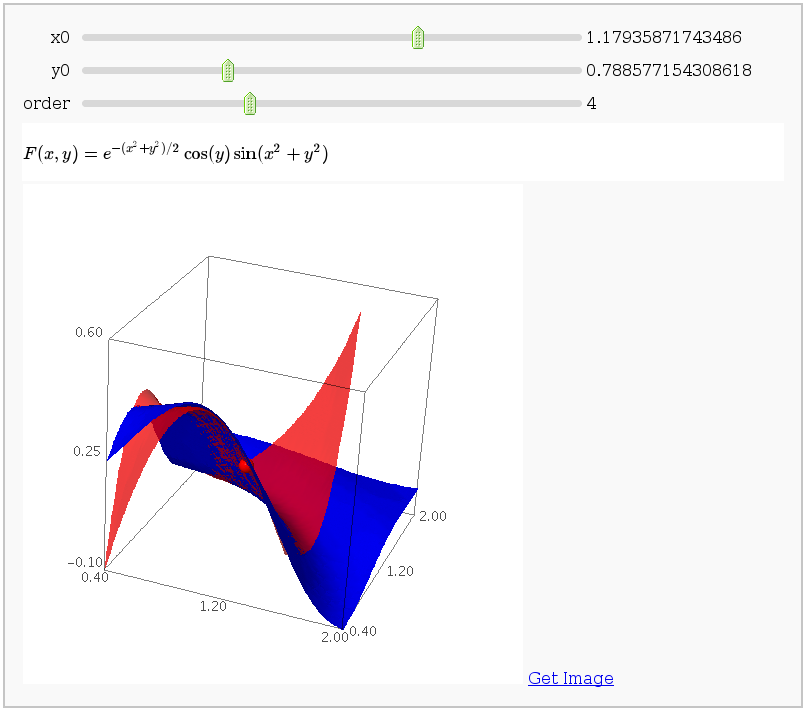
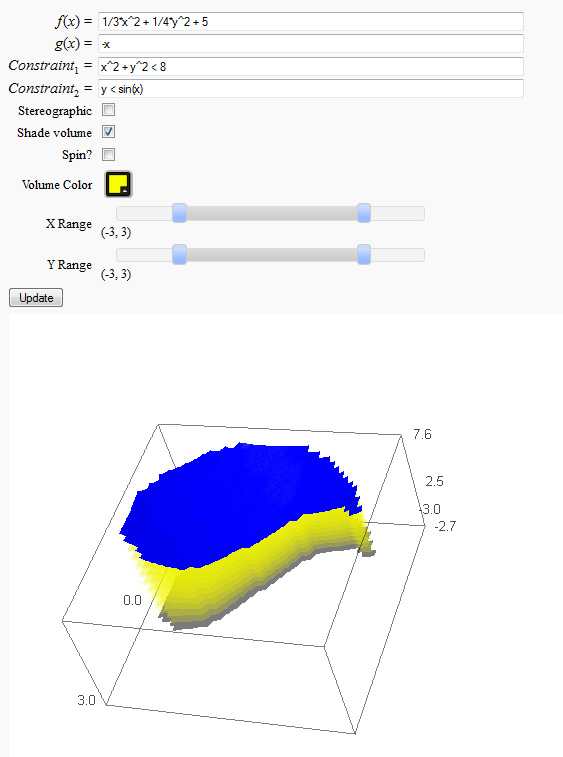
Volumes over non-rectangular domains

by John Travis

https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2829.sagews

3D_Irregular_Volume.png

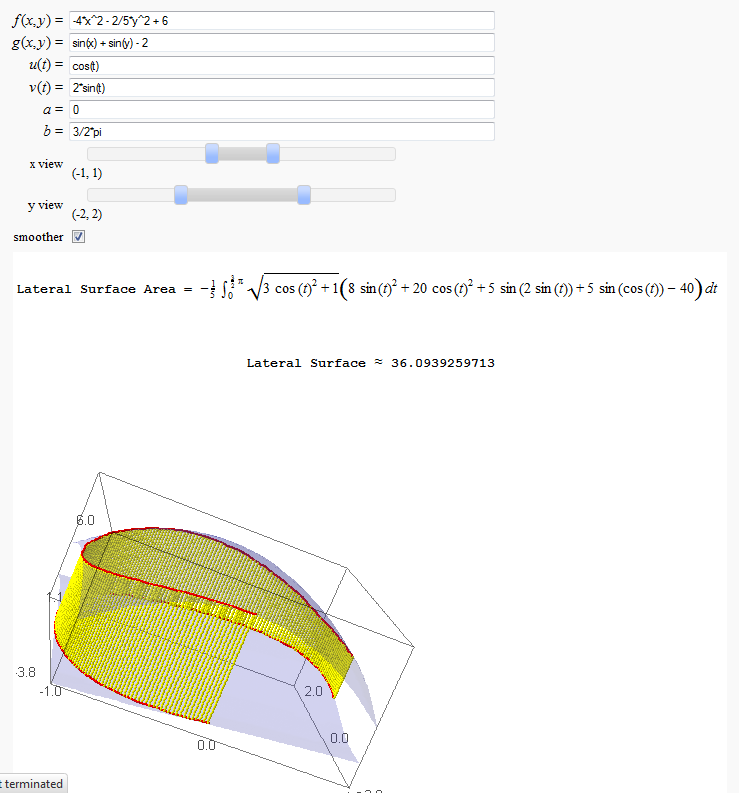
Lateral Surface Area (FIXME in Jupyter)

by John Travis

http://sagenb.mc.edu/home/pub/89/

Lateral_Surface.png

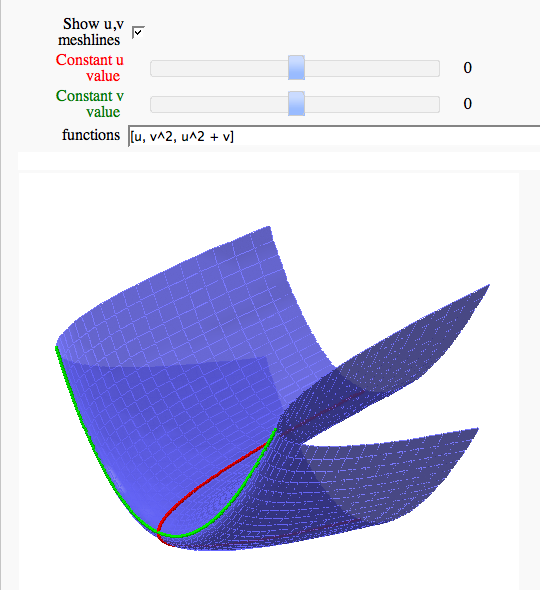
Parametric surface example (FIXME in Jupyter)

by Marshall Hampton

parametric_surface.png

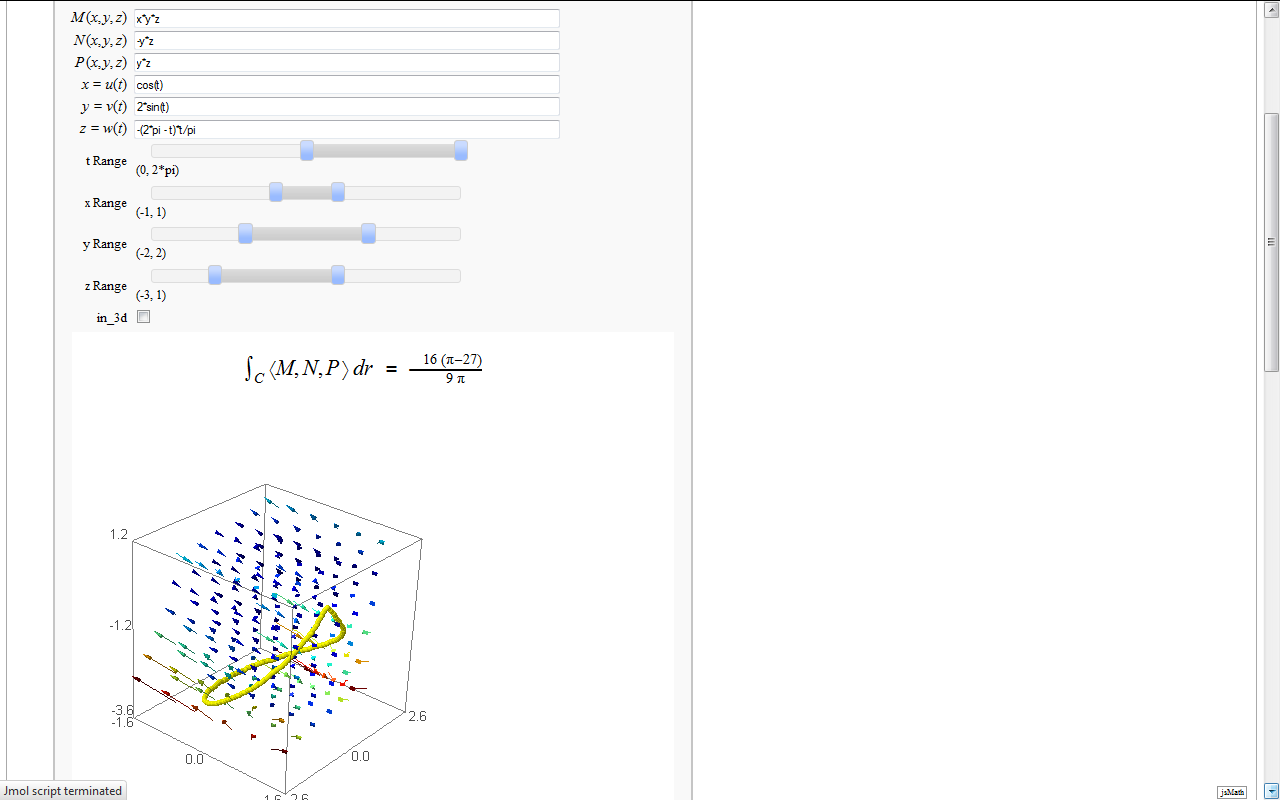
Line Integrals in 3D Vector Field

by John Travis

https://cloud.sagemath.com/projects/19575ea0-317e-402b-be57-368d04c113db/files/pub/2801-2901/2827-$%20%5Cint_%7BC%7D%20%5Cleft%20%5Clangle%20M,N,P%20%5Cright%20%5Crangle%20dr%20$%20=%20$%20%25s%20$.sagews

3D_Line_Integral.png

interact/calculus (last edited 2020-08-11 14:10:09 by kcrisman)