Sage Interactions - Geometry

goto interact main page

Intersecting tetrahedral reflections FIXME

by Marshall Hampton. Inspired by a question from Hans Schepker of Glass Geometry.

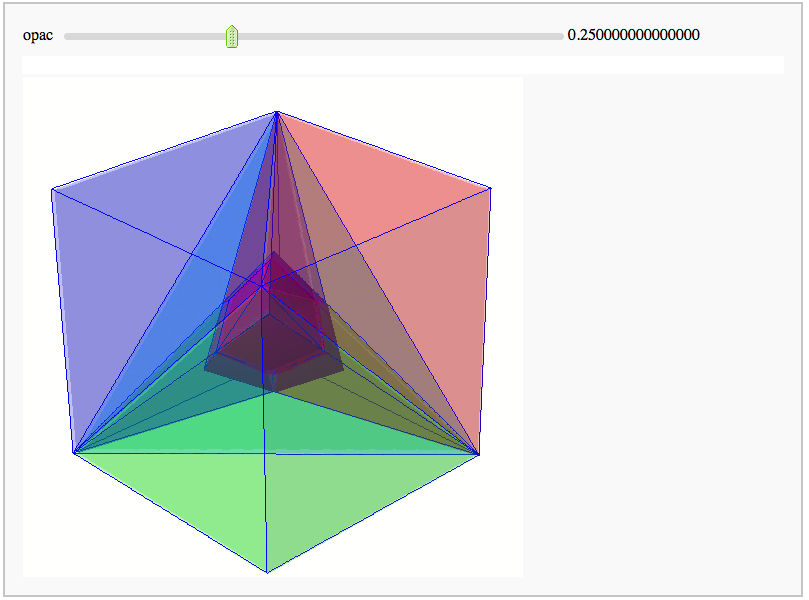
Evolutes

by Pablo Angulo. Computes the evolute of a plane curve given in parametric coordinates. The curve must be parametrized from the interval [0,2pi].

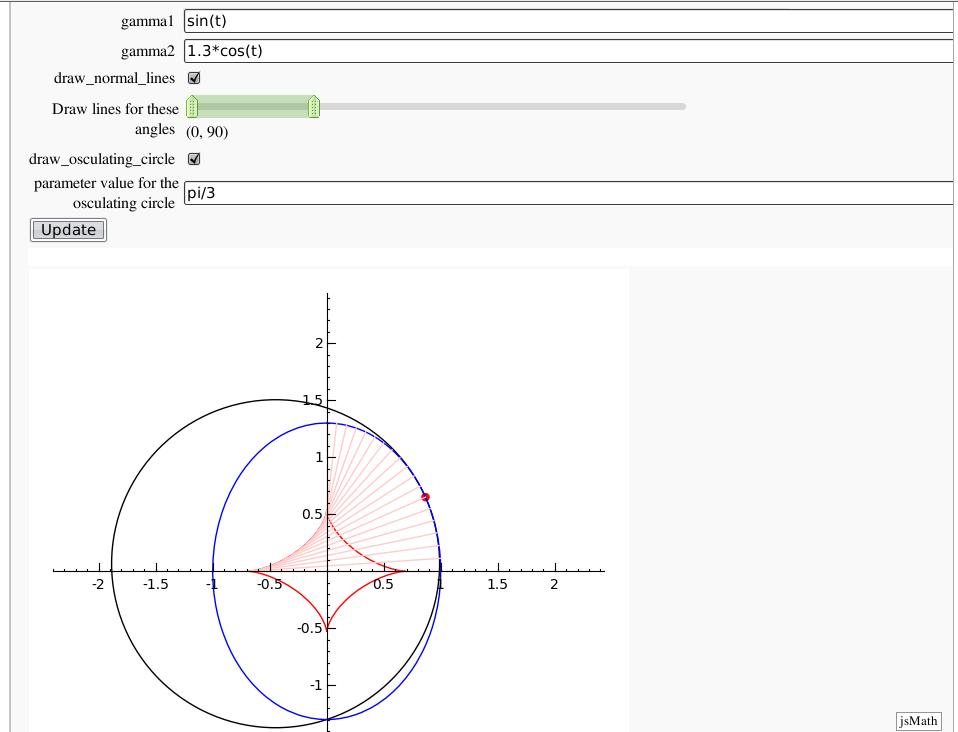
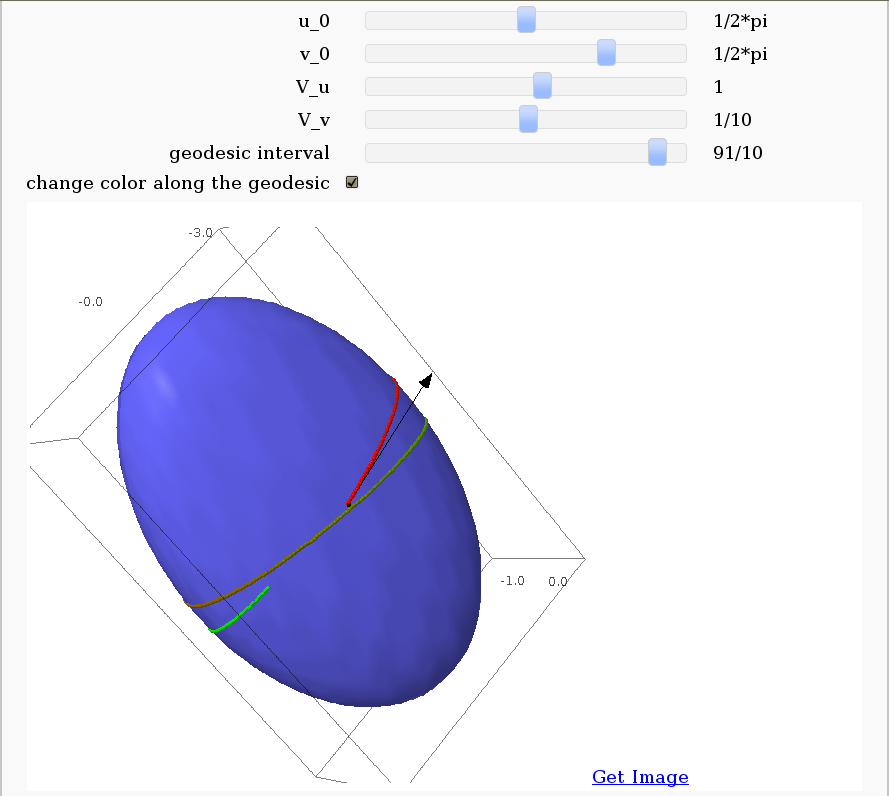
Geodesics on a parametric surface

by Antonio Valdés and Pablo Angulo. This example was originally composed of two interacts:

• - the first allowing the user to introduce a parametric surface, and draw it. - the second drawing a geodesic within the surface.

The separation was so that after the first interact, the geodesic equations were "compiled", thus making the second interact faster. However, in the following there is only one interact, to make sagecell works.

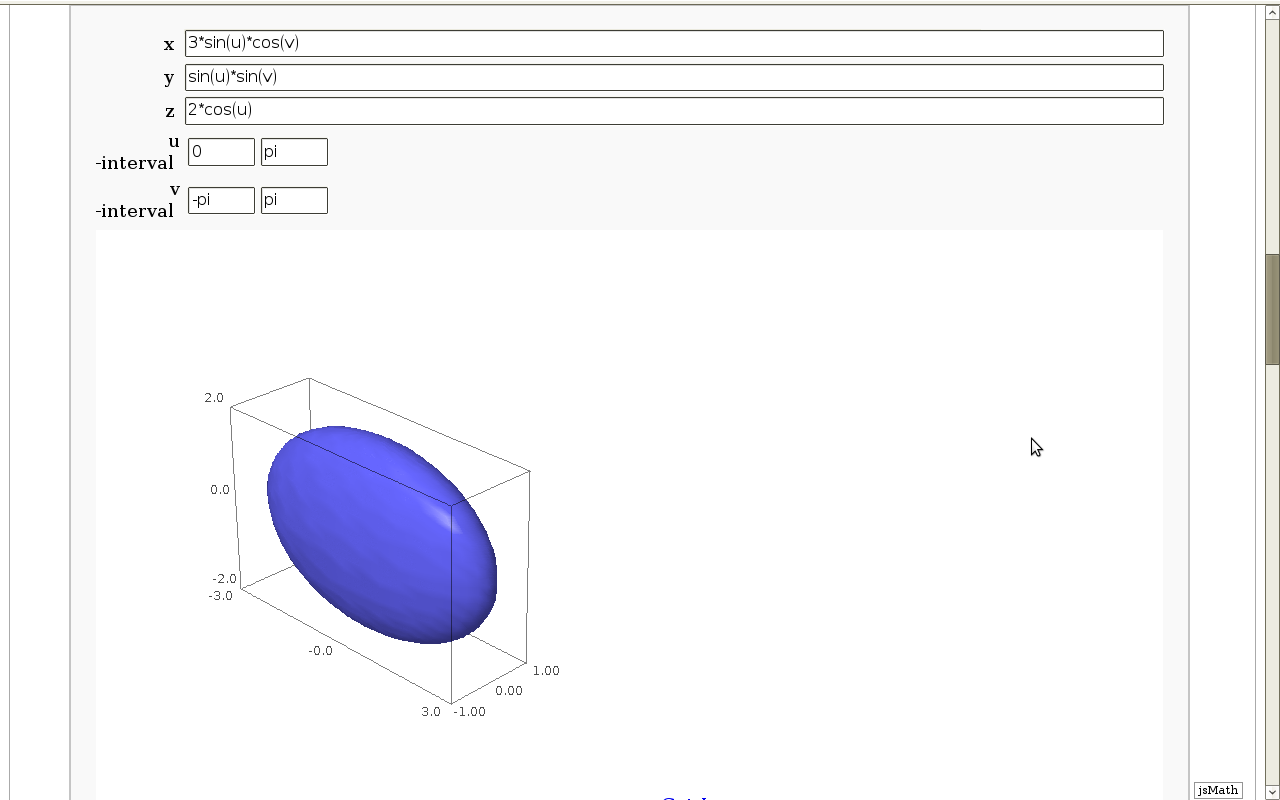
Dimensional Explorer

By Eviatar Bach

Renders 2D images (perspective or spring-layout) and 3D models of 0-10 dimensional hypercubes. It also displays number of edges and vertices.

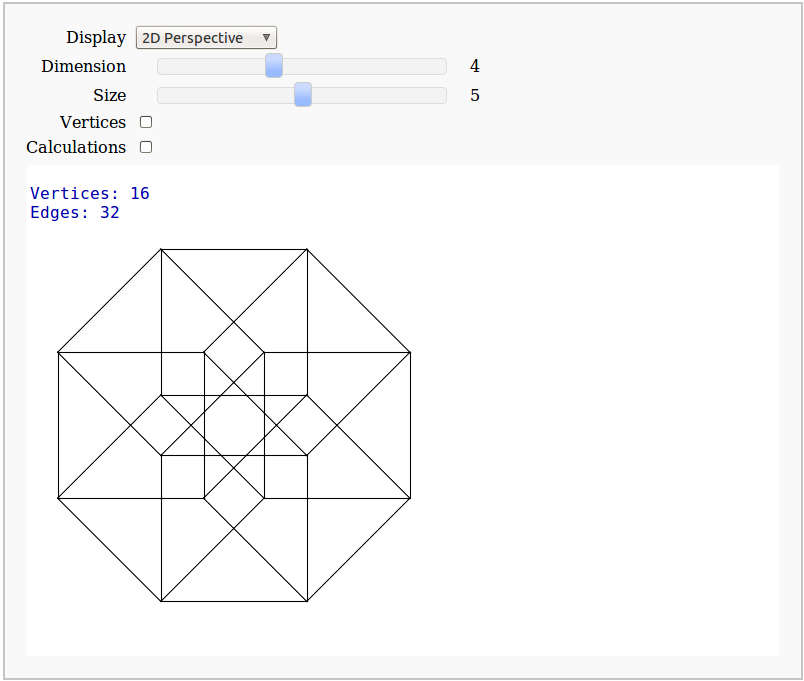
Crofton's formula

by Pablo Angulo. Illustrates Crofton's formula by throwing some random lines and computing the intersection number with a given curve. May use either solve for exact computation of the intersections, or may also approximate the curve by straight segments (this is the default).

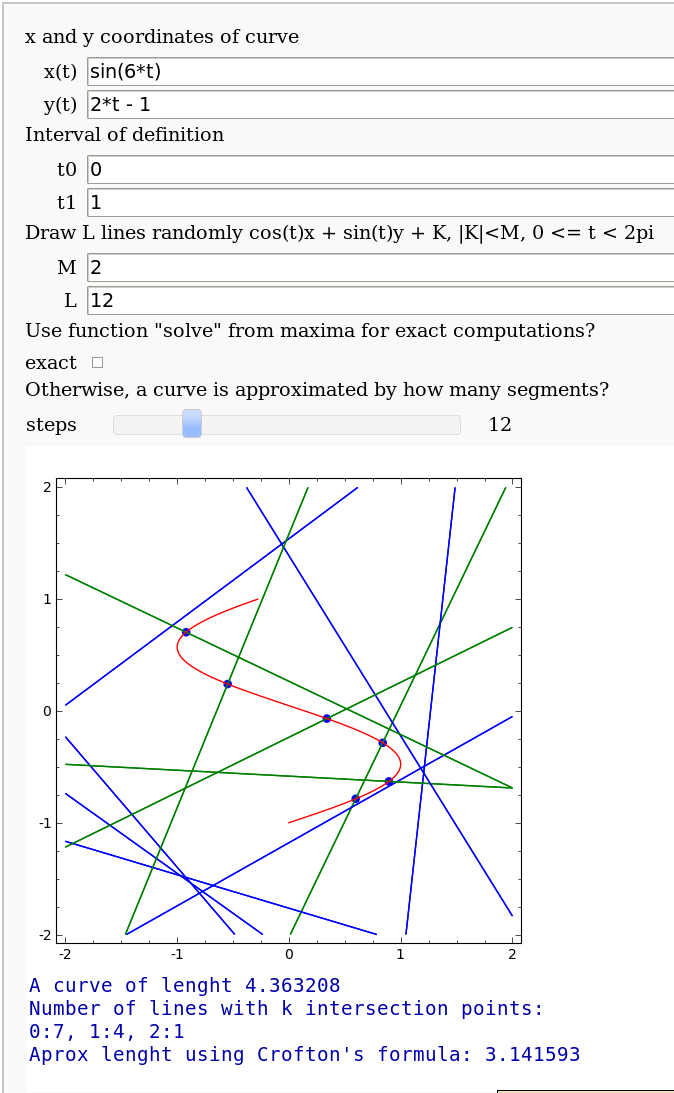
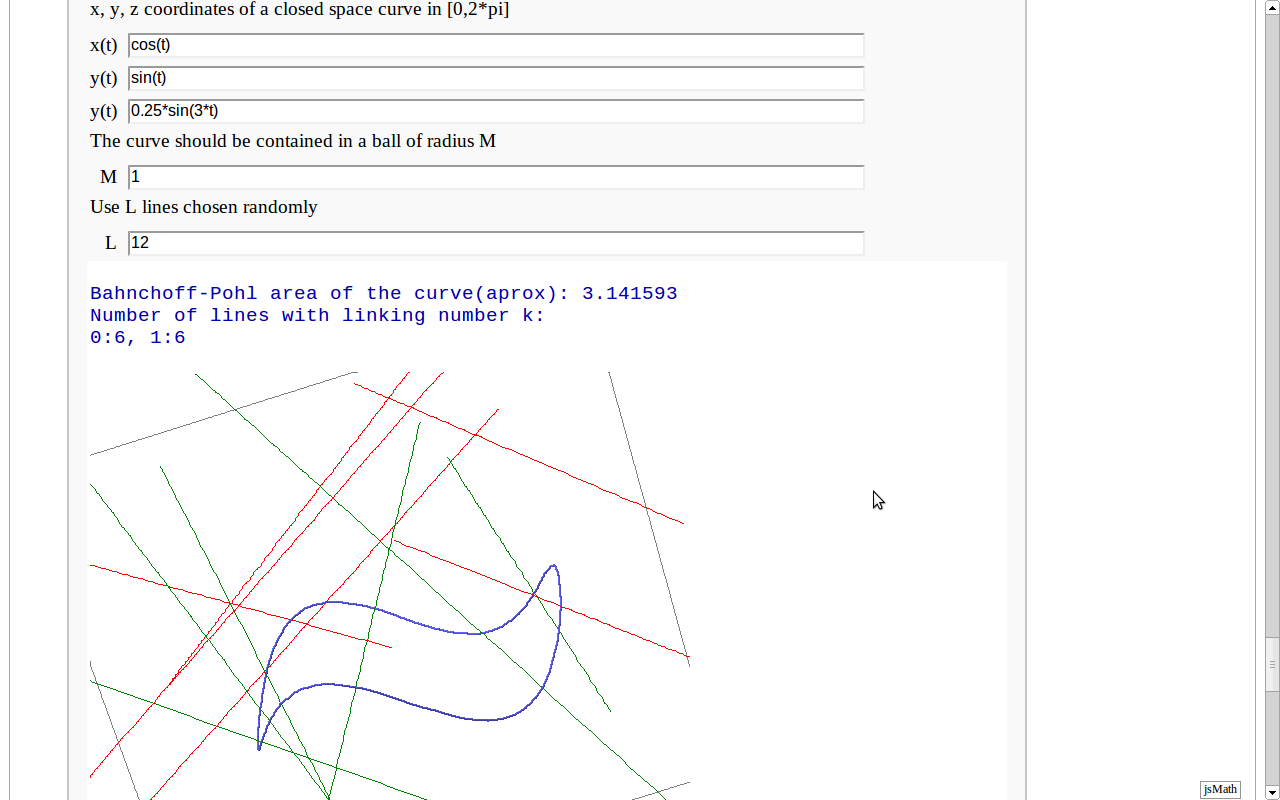
Banchoff-Pohl "area"

by Pablo Angulo. Approximates the Banchoff-Pohl "area enclosed by a spatial curve", by throwing some random lines and computing the linking number with the given curve. Lines not linked to the given curve are displayed in red, linked lines are displayed in green.